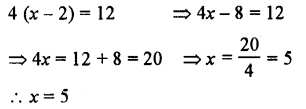Selina Concise Mathematics Class 7 ICSE Solutions Chapter 12 Simple Linear Equations

Selina Concise Mathematics Class 7 ICSE Solutions Chapter 12 Simple Linear Equations

Selina Concise Mathematics Class 7 ICSE Solutions Chapter 12 Simple Linear Equations (Including Word Problems)

Selina Publishers Concise Maths Class 7 ICSE Solutions Chapter 12 Simple Linear Equations (Including Word Problems)

POINTS TO REMEMBER

1. Equation: An equation is a statement which states that two expressions are equal.

2. To solve an equation means to find the value of the variable (unknown quantity) used in it.

Note : An equation remains unchanged if
(i) the same number is added to each side of the equation. .
(ii) the same number is subtracted from each side of the equation.
(iii) the same number is multiplied to each side of the equation.
(iv) Each side of the equation is divided by the same non-zero number.

(v) In transposing any term of an equation from one side to another, then its sign is reversed is

(a) from positive to negative and from negative to positive

(b) from multiplication to division and from division to multiplication.
3. In equation :

It is a statement of inequality between two expressions involving a single variable with the highest power one.

4. Replacement set

For a given inequation, the set from which the values of its variable are taken is called the replacement set or domain of the variable.
5. Solution set

It is the subset of the replacement set, consisting of those values of the variable which satisfy the given inequation

6. Properties of inequations

Adding, subtracting, multiplying or dividing by the same positive number to each side of an inequation does not change the inequality but multiplying or dividing by a negative number to each side of an inequation, it changes the inequality.

Simple Linear Equations Exercise 12A – Selina Concise Mathematics Class 7 ICSE Solutions

Solve the following equations :

Question 1.
x + 5 = 10

Solution:
x + 5 = 10
⇒ x=10 -5 = 5

Question 2.
2 + y=7

Solution:
2 + y = 7
⇒ = 7- 2 = 5

Question 3.
a – 2 = 6

Solution:
a -2 =6
⇒a = 6 + 2 = 8

Question 4.
x – 5 = 8

Solution:
x-5 =8
⇒ x = 8 +5 = 13

Question 5.
5 – d= 12

Solution:
5-d = 12
⇒ -d = 12-5 =7
⇒ d = – 7

Question 6.
3p = 12

Solution:
3p = 12
⇒ P == 4 Ans.

Question 7.
14 = 7m

Solution:
14 = 7m
⇒ m == 2

Question 8.
2x = 0

Solution:
2x = 0 ⇒ x == 0

Question 9.

= 2

Solution:

= 2
⇒x = 2 ×9 = 18
∴ x = 18

Question 10.

= -4

Solution:

 = -4
⇒= -4
⇒ y = (-4) × (-12)
∴ y= 48

Question 11.
8x-2 =38

Solution:
8x-2 =38
8x = 38 + 2 = 40
⇒ x = = 5
∴ x = 5

Question 12.
2x + 5 = 5

Solution:
2x + 5 = 5
⇒ 2x = 5 – 5 = 0
x == 0
∴x = 0

Question 13.
5x – 1 = 74

Solution:
5x- 1 = 74
⇒ 5x = 74 + 1 = 75
⇒ x == 15

Question 14.
14 = 27-x

Solution:
14 = 27 -x
⇒ x = 27- 14
⇒ x = 13
∴ x= 13

Question 15.
10 + 6a = 40

Solution:
10 + 6a = 40
⇒ 6a = 40 -10 = 30
⇒ a == 5
∴ a= 5

Question 16.

Solution:
 


Question 17.
 

Solution:
 


Question 18.
12 = c – 2

Solution:
12 = c – 2
⇒ 12 + 2 =c
⇒ 14 = c
∴c = 14

Question 19.
4 = x- 2.5

Solution:
4 = x – 2.5
⇒4 + 2.5=x
⇒ 6.5 =x
∴ x = 6.5

Question 20.
 
Solution:
 

Question 21.

Solution:

 Question 22.
p + 0.02 = 0.08

Solution:
p + 0.02 = 0.08
⇒ p = 0.08 – 0.02 = 0.06
∴ p = 0 06

Question 23.

Solution:
 

Question 24.


Solution:
 

Question 25.
Solution:


Question 26.


Solution:

 Question 27.
 

Solution:

 


Question 28.
2a – 3 =5

Solution:
2a – 3 = 5
⇒2a = 5 +3
⇒ 2a = 8
⇒ a == 4
∴a = 4

Question 29.
3p – 1 = 8

Solution:
3p – 1 = 8
⇒3p = 8 + 1 = 9
⇒ p == 3
∴p = 3

Question 30.
9y -7 = 20

Solution:
 

Question 31.
2b – 14 = 8

Solution:

 

Question 32.


Solution:
 

Question 33.

Solution:
 

Simple Linear Equations Exercise 12B – Selina Concise Mathematics Class 7 ICSE Solutions

Question 1.
8y – 4y = 20

Solution:
 

Question 2.
9b – 4b + 3b = 16

Solution:
 

Question 3.
5y + 8 = 8y – 18

Solution:
 

Question 4.
6 = 7 + 2p -5

Solution:
 

Question 5.
8 – 7x = 13x + 8

Solution:
 

Question 6.
4x – 5x + 2x  = 28 + 3x

Solution:
 

Question 7.
9 + m = 6m + 8 – m

Solution:
 

Question 8.
24 = y + 2y + 3 + 4y

Solution:
 

Question 9.
19x -+ 13 -12x + 3 = 23

Solution:
 

Question 10.
6b + 40 = – 100 – b

Solution:
 

Question 11.
6 – 5m – 1 + 3m = 0 

Solution:
 

Question 12.
0.4x – 1.2  = 0.3x + 0.6

Solution:
 

Question 13.
6(x+4) = 36

Solution:
 

Question 14.
9 ( a+ 5) + 2 = 11

Solution:
 

Question 15.
4 ( x- 2 ) = 12

Solution:
 

Question 16.
-3 (a- 6 ) = 24

Solution:
 

Question 17.
7 ( x-2) = 2 (2x -4)

Solution:
 

Question 18.
(x-4) (2x +3 ) = 2x²

Solution:
 

Question 19.

21 – 3 ( b-7 ) = b+ 20

Solution:
 

Question 20.
x (x +5 ) = x² +x + 32

Solution:
 

Simple Linear Equations Exercise 12C – Selina Concise Mathematics Class 7 ICSE Solutions

Solve

Question 1.
 

Solution:
 

Question 2.
 

Solution:
 

Question 3.

 Solution:
 

Question 4.
 

Solution:
 

Question 5.
 

Solution:
 

Question 6.
 

Solution:
 

Question 7.
 

Solution:
 

Question 8.

 Solution:
 

Question 9.
 

Solution:
 

Question 10.
 

Solution:
 

Question 11.

 Solution:

 
Question 12.
0.6a +0.2a = 0.4 a +8

Solution:
 

Question 13.
p + 104p= 48

Solution:
 

Question 14.
10% of x = 20

Solution:
 

Question 15.
y + 20% of y = 18

Solution:
 

Question 16.
x – 13% of x = 35

Solution:
 

Question 17.

 Solution:
 

Question 18.

Solution:
 

Question 19.

 Solution:
 

Question 20.
 

Solution:

 

Question 21.
 

Solution:

Question 22.
 

Solution:
 

Question 23.
15 – 2 (5-3x ) = 4 ( x-3 ) + 13

Solution:
 

Question 24.
 

Solution:
 

Question 25.
21 – 3 (x – 7) = x + 20

Solution:
 

Question 26.
 

Solution:
 

Question 27.
 

Solution:
 

Question 28.
 

Solution:
 

Question 29.
 

Solution:
 

Question 30.
2x + 20% of x = 12.1

Solution:
 

Simple Linear Equations Exercise 12D – Selina Concise Mathematics Class 7 ICSE Solutions

Question 1.
One-fifth of a number is 5, find the number.

Solution:
Let the number = x
According to the condition
 x = 5 ⇒ x = 5 x 5
⇒ x = 25
∴ Number = 25

Question 2.
Six times a number is 72, find the number.

Solution:
Let the number = x
According to the condition
6x = 72
⇒ x =
⇒x= 12
∴ Number = 12

Question 3.
If 15 is added to a number, the result is 69, find the number.

Solution:
Let the number = x
According to the condition
x+ 15 = 69
⇒ x = 69 – 15 x = 54
∴Number = 54

Question 4.
The sum of twice a number and 4 is 80, find the number.

Solution:
Let the number = x
According to the condition
2x + 4 = 80
⇒2x = 80 – 4
⇒ 2x = 76
⇒ x == 38
Number = 38

Question 5.
The difference between a number and one- fourth of itself is 24, find the number.

Solution:
 

Question 6.
Find a number whose one-third part exceeds its one-fifth part by 20.

Solution:
 

 

Question 7.
A number is as much greater than 35 as is less than 53. Find the number.

Solution:
Let the number = x
According to the condition
x – 35 = 53 – x
⇒ x + x = 53 + 35
88
⇒2x = 88
⇒ x == 44
∴Number = 44

Question 8.
The sum of two numbers is 18. If one is twice the other, find the numbers.

Solution:
Let the first number = x
and the second number = y
According to the condition
x + y= 18 …(i)
and x = 27 ….(ii)
Substitute the eq. (ii) in eq. (i), we get
2y + y= 18
x= 2y = 18
⇒ 3y= 18 ⇒y== 6
Now, substitute the value of y in eq. (ii), we get
x = 2 x 6= 12
∴ The two numbers are 12, 6

Question 9.
A number is 15 more than the other. The sum of of the two numbers is 195. Find the numbers.

Solution:
Let the First number = x
and the Second number = y
According to the condition
x = y+ 15 …(i)
x + 7=195 …(ii)
Substitute the eq. (i) in eq. (ii), we get
y+15+7=195
⇒2y= 195- 15
⇒ y = = 90
Now, substitute the value of y in eq. (i), we get
x = 90+ 15 = 105
∴ The two numbers are 105 and 90

Question 10.
The sum of three consecutive even numbers is 54. Find the numbers.

Solution:
Let the first even number = x
second even number = x + 2
and third even number = x + 4
According to the condition,
x + x + 2 + x + 4 = 54
⇒ 3x + 6 = 54
⇒ 3x = 54 – 6
⇒ x == 16
∴ First even number = 16
Second even number = 16 + 2 = 18
and third even number = 16 + 4 = 20

Question 11.
The sum of three consecutive odd numbers is 63. Find the numbers.

Solution:
Let the first odd number = x
second odd number = x + 2
and third odd number = x + 4
According to the condition,
x+ x + 2 + x+4 = 63
3x + 6 = 63 ⇒ 3x = 63 – 6
⇒3x = 57 ⇒ x ==19
∴ First odd number = 19
Second odd number = 19 + 2 = 21
third odd number = 19 + 4 = 23

Question 12.
A man has ₹ x from which he spends ₹6. If twice of the money left with him is ₹86, find x.

Solution:
Let the total amount be x
According to the condition
2x = 86
⇒x =
⇒ x = 43
Amount spent by him = 6
∴Total money he have = ₹43 + ₹6 = ₹49

Question 13.
A man is four times as old as his son. After 20 years, he will be twice as old as his son at that time. Find their present ages.

Solution:
Let the present age of the son = x years
Present age of the father = 4x years
After 20 years,
Son’s age will be (x + 20) years
and Father’s age will be (4x + 20) years
According to the condition,
4x + 20 = 2 (x + 20)
4x + 20 = 2x + 40
4x – 2x = 40 – 20
2x = 20
⇒ x = 10

∴Present age of the son = 10 years and Present age of the father = 4×10 years = 40 years

Question 14.

If 5 is subtracted from three times a number, the result is 16. Find the number.

Solution:
Let the number = x
According to the condition,
3x – 5 = 16
⇒ 3x = 16 + 5
⇒ 3x = 21
⇒ x =
⇒ x = 7
∴The number = 7

Question 15.
Find three consecutive natural numbers such that the sum of the first and the second is 15 more than the third.

Solution:
Let the first conscutive number = x,
Second consecutive number = x + 1
and Third consecutive number = x + 2
According to the condition,
x + x + 1 = 15 + x + 2
⇒ 2x + 1 = 17 +x
⇒ 2x -x = 17 – 1
⇒ x= 16
∴ The first consecutive number = 16
Second consecutive number =16+1 = 17
Third consecutive number =16 + 2=18

Question 16.
The difference between two numbers is 7. Six times the smaller plus the larger is 77. Find the numbers.

Solution:
Let the smallest number = x
and the largest number = y
According to the condition,
y-x = 7 …(i)
and 6x + y = 77 ….(ii)
From eq. (i)
y = 7 + x …(iii)
Substitute the eq. (iii) in eq. (ii)
6x + 7 + x = 77
⇒ 7x = 77-7
⇒ x == 10
Now, substitute the value of x in eq. (iii)
y = 7+ 10= 17
∴The smallest number 10 and the largest number is 17.

Question 17.
The length of a rectangular plot exceeds its breadth by 5 metre. If the perimeter of the plot is 142 metres, find the length and the breadth of the plot.

Solution:
 

Question 18.
The numerator of a fraction is four less than its denominator. If 1 is added to both, is numerator and denominator, the fraction becomesFind the fraction.

Solution:

 
Question 19.
A man is thrice as old as his son. After 12 years, he will be twice as old as his son at that time. Find their present ages.

Solution:
Let the present age of the son = x years
and the present age of the father = 3x years
After 12 years,
Son’s age will be (x + 12) years
and father’s age will be (3x + 12) years
According to the condition,
3x + 12 = 2 (x + 12)
3x + 12 = 2x+ 24
3x – 2x = 24 – 12
x= 12
∴Present age of the son = 12 years
and Present age of the father = 3×12 years
= 36 years

Question 20.
A sum of ₹ 500 is in the form of notes of denominations of ₹ 5 and₹ 10. If the total number of notes is 90, find the number of notes of each type.

Solution:
Let the number of ₹ 5 notes = x
∴ The number of ₹10 notes = 90 – x
Value of ₹10 notes = x ×₹ 5 = ₹3x
and value of ₹10 notes = (90 – x) x ₹ 10 =₹(900 – 10x)
∴Total value of all the notes = ₹500
∴5x+ (900- 10x) = 500
⇒ 5x + 900 – 10x = 500
⇒ -5x = 500 – 900
⇒ x =
⇒ x = 80
∴ The number of ₹5 notes = x = 80
and the number of ₹10 notes = 90 – x
= 90 – 80= 10