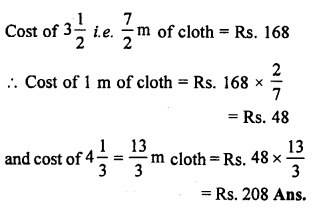Selina Concise Mathematics class 7 ICSE Solutions Chapter 7 – Unitary Method (Including Time and Work)

Selina Concise Mathematics class 7 ICSE Solutions – Unitary Method (Including Time and Work)

Selina Concise Mathematics class 7 ICSE Solutions – Unitary Method (Including Time and Work)

The method in which the value of a unit (one) quantity is first calculated to get the value of any other quantity is called the unitary method.

In unitary method, we come across two types of variations :

(i) Direct-variation

(ii) Inverse-variation.

(i) Direct variation : Increase in one quantity causes increase in the other and decrease in one quantity causes decrease in the other.

(ii) Inverse variation : Increase in one quantity causes decrease in the other and decrease in one quantity causes increase in the other.

This is found in the sums of speed, work done etc.

EXERCISE 7 (A)

Question 1.
Weight of 8 identical articles is 4.8 kg. What is the weight of 11 such articles ?

Answer:
Weight of 8 articles = 4.8 kg
Weight of 1 article =  kg
and weight of 11 articles =   x 11 kg

= 0.6 x 11 = 6.6 kg

Question 2.
6 books weigh 1 .260 kg. How many books will weigh 3.150 kg ?
Answer:
 

Question 3.
8 men complete a work in 6 hours. In how many hours will 12 men complete the same work ?

Answer:
8 men can complete a work in = 6 hours
1 man can complete the work in = 6×8 hours
12 men can complete the work in =  = 4 hours

Question 4.
If a 25 cm long candle burns for 45 minutes, how long will another candle of the same material and same thickness but 5 cm longer than the previous one, burn ?

Answer:
25 cm long candle burn in = 45 minutes
1 cm long candle will burn in =  mintues
25 + 5 = 30 cm long candle will burn in
= minutes = 54 minutes

Question 5.
A typist takes 80 minutes to type 24 pages. How long will he take to type 87 pages ?

Answer:
For typing 24 pages, time is required = 80 minutes
For typing 1 page, time is required =  minutes
and for typing 87 pages, time is required
= minutes = 290 minutes

Question 6.
Rs. 750 support a family for 15 days. For how many days will Rs. 2,500 support the same family ?

Answer:
Rs. 750, can support a family for = 15 days
Re. 1 will support for = days
and Rs. 2,500 will support for =   x 2500 days = 50 days

Question 7.
400 men have provisions for 23 weeks. They are joined by 60 men. How long will the provisions last ?

Answer:
400 men have provisions for = 23 weeks
1 man will have provisions for = 23 x 400 weeks
and 400 + 60 = 460 men will have provisions for = weeks = 20weeks

Question 8.
200 men have provisions for 30 days. If 50 men left, the same provisions would last for the remaining men, in how many days?

Answer:
200 men have provisions for = 30 days
1 man will have provisions for = 30 x 200 days
200 – 50 = 150 men will have provisions
for = days = 40 days

Question 9.
8 men can finish a certain amount of provisions in 40 days. If 2 more men join with them, find for how many days the same amount of provisions be sufficient ?

Answer:
8 men can finish a provision in = 40 days
1 man will finish in = 40 x 8 days
8+2=10 men will finish in = = 32 days

Question 10.
If interest on Rs. 200 be Rs. 25 in a certain time, what will be the interest on Rs 750 for the same time ?

Answer:
 

 

Question 11.
If 3 dozen eggs cost Rs. 90, find the cost of 3 scores of eggs. (1 score = 20)

Answer:
3 dozen = 3 x 12 = 36 eggs,
3 scores = 3 x 20 = 60
The cost of 36 eggs is = Rs. 90
The cost of 1 egg will be = Rs. 

∴ Cost of 60 eggs will be = Rs. 
= Rs. 150

Question 12.
If the fare for 48 km is Rs. 288, what will be the fare for 36 km ?
Answer:
Fare for 48 km = Rs. 288
fare for 1 km = Rs. = Rs. 216

Question 13.
What will be the cost of 3.20 kg of an item, if 3 kg of it costs Rs. 360 ?
Answer:
 

Question 14.
If 9 lines of a print, in a column of a book contains 36 words. How many words will a column of 51 lines cqntain ?

Answer:
 

Question 15.

125 pupil have food sufficient for 18 days. If 25 more pupil join them, how long will the food last now ? What assumption have you made to come to your answer ?

Answer:

Pupils in the beginning = 125
More pupils joined = 25
Total pupils = 125 + 25 = 150
Food is sufficient for 125 pupils for = 18 days
Food will be sufficient for 1 pupil for = 18 x 125 days (less pupil more days)
and food will be sufficient for 150 pupils = days (more pupil more days)
=  =15 days

Question 16.
A carpenter prepares a new chair in 3 days, working 8 hours a day. Atleast how many hours per day must he work in order to make the same chair in 4 days ?

Answer:
A chair is completed in 3 days working per day = 8 hours
Then their will be completed in 1 day working for = 8 x 3 hours per day (less days more hours)
and it will be completed in 4 days working for = = 6 hours per day.

Question 17.
A man earns ₹5,800 in 10 days. How much will he earn in the month of February of a leap year?
Answer:

 

Question 18.
A machine is used for making rubber balls and makes 500 balls in 30 minutes. How many balls will it make in 3  hours?
Answer:
 

Question 19.
In a school’s hostel mess, 20 children consume a certain quantity of ration in 6 days. However, 5 children did not return to the hostel after holidays. How long will the same amount of ration last now?

Answer:
Total number of children = 20
20 children consume a certain quantity of ration in = 6 days
1 children consume a certain quantity of ration in = 6 x 20 days
As 5 children did not return to the hostel after holidays.
Then number of children in hostel = 20-5 = 15
Hence, 15 children consume certain quantity 6×20
of ration in =  days = 8 days

EXERCISE 7 (B)

Question 1.
 

Answer:
 

Question 2.
3 m of cloth costs Rs. 168 ; find the cost of 4 m of the same cloth.
Answer:
 

Question 3.
A wrist watch loses 10 sec in every 8 hours; in how much time will it lose 15 sec. ?

Answer:
 

Question 4.
In 2 days and 20 hours, a watch gains 20 sec ; find how much time will the watch take to gain 35 sec. ?

Answer:
 

Question 5.
50 men mow 32 hectares of land in 3 days. How many days will 15 men take to mow it?

Answer:
Land is same in both the cases.
Now 50 men can mow land in = 3 days
∴ 1 man will mow it in = 3 x 50 days
and 15 men will mow it in =  = 10 days

Question 6.
The wages of 10 workers for a six days week are Rs, 1,200. What are the one day wages: (i) of one worker ? (ii) of 4 workers?

Answer:
 

Question 7.
If 32 apples weigh 2 kg 800 g. How many apples will there be in a box, containing 35 kg of apples ?

Answer:
 

Question 8.
A truck uses 20 litres of diesel for 240 km. How many litres will be needed for 1200 km?

Answer:
For 240 km, diesel is needed = 20 litres
∴ for 1 km, diesel will be needed 20

Question 9.
A garrison of 1200 men has provisions for 15 days. How long will the provisions last if the garrison be increased by 600 men ?

Answer:
1200 men has provision for = 15 days
1 man will have that provision for = 15 x 1200 days
∴1200 + 600 = 1800 men will has that provisions for =  days

= 10 days

Question 10.
A camp has provisions for 60 pupil for 18 days. In how many days, the same provisions will finish off if the strength of the camp is increased to 72 pupil ?

Answer:

60 pupil have provision for = 18 days 1 pupil will have provision for = 18 x 60 days (less pupils more days)

and 72 pupils will have provision for = days

= 15 days.

EXERCISE 7 (C)

Question 1.
A can do a piece of work in 6 days and B can do it in 8 days. How long will they take to complete it together ?

Answer:
 

Question 2.
A and B working together can do a piece of work in 10 days B alone can do the same work in 15 days. How long will A alone take to do the same work ?

Answer:
 

Question 3.
A can do a piece of work in 4 days and B can do the same work in 5 days. Find, how much work can be done by them working together in : (i) one day (ii) 2 days.
What part of work will be left, after they have worked together for 2 days ?

Answer:
 

Question 4.
A and B take 6 hours and 9 hours respectively to complete a work. A works for 1 hour and then B works for two hours.
(i) How much work is done in these 3 hours ?
(ii) How much work is still left ?

Answer:
 

Question 5.
A, B and C can do a piece of work in 12, 15 and 20 days respectively. How long will they take to do it working together ?

Answer:
 

Question 6.
Two taps can fill a cistern in 10 hours and 8 hours respectively. A third tap can empty it in 15 hours. How long will it take to fill the empty cistern, if all of them are opened together ?

Answer:
 

Question 7.
Mohit can complete a work in 50 days, whereas Anuj can complete the same work in 40 days.
Find:
(i) work done by Mohit in 20 days.
(ii) work left after Mohit has worked on it for 20 days.
(iii) time taken by Anuj to complete the remaining work.

Answer:
 

 

Question 8.
Joseph and Peter can complete a work in 20 hours and 25 hours respectively.
Find :
(i) work done by both together in 4 hrs.
(ii) work left after both worked together for 4 hrs.
(iii) time taken by Peter to complete the remaining work.

Answer:
 

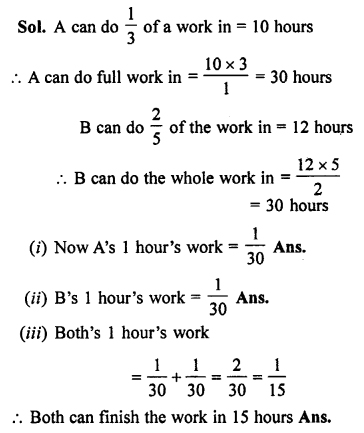
Question 9.
A is able to complete of a certain work in 10 hrs and B is able to complete  of the same work in 12 hrs.
Find:
(i) how much work can A do in 1 hour ?
(ii) how much work can B do in 1 hour ?
(iii) in how much time will the work be completed, if both work together.

Answer:
 

Question 10.
Shaheed can prepare one wooden chair in 3 days and Shaif can prepare the same chair in 4 days. If they work together, in how many days will they prepare :
(i) one chair ?
(ii)14 chairs of the same kind?

Answer:
 

Question 11.
A, B and C together finish a work in 4 days. If A alone can finish the same work in 8 days and B in 12 days, find how long will C take to finish the work.

Answer: