Selina Concise Physics Class 10 ICSE Solutions Force
Selina ICSE Solutions for Class 10 Physics Chapter 1 Force
Exercise 1(A)
1. State the condition when on applying a force, the body has:
(a) the translational motion,
(b) the rotational motion.
(a) the translational motion,
(b) the rotational motion.
Solution 1.
(a) When the body is free to move it produces translational motion.
(b) When the body is pivoted at a point, it produces rotational motion.
2. Define moment of force and state its S.I. unit.
2. Define moment of force and state its S.I. unit.
Solution 2.
The moment of force is equal to the product of the magnitude of the force and the perpendicular distance of the line of action of force from the axis of rotation.
S.I. unit of moment of force is Newton metre (Nm).
3. State whether the moment of force is a scalar or vector quantity?
3. State whether the moment of force is a scalar or vector quantity?
Solution 3.
Moment of a force is a vector.
4. State two factors affecting the turning effect of a force.
4. State two factors affecting the turning effect of a force.
Solution 4.
Moment of force about a point depends on the following two factors:
- The magnitude of the force applied and,
- The distance of line of action of the force from the axis of rotation.
5. When does a body rotate? State one way to change the direction of rotation of a body. Give a suitable example to explain your answer.
Solution 5.
When the body is pivoted at a point, the force applied on the body at a suitable point rotates the body about the axis passing through the pivoted point.
The direction of rotation can be changed by changing the point of application of force. The given figure shows the anticlockwise and clockwise moments produced in a disc pivoted at its centre by changing the point of application of force F from A to B.
Solution 6.
Moment of force about a given axis = force x perpendicular distance of force from the axis of rotation.
7. State one way to reduce the moment of a given force about a given axis of rotation.
7. State one way to reduce the moment of a given force about a given axis of rotation.
Solution 7.
Moment of force depends on the distance of line of action of the force from the axis of rotation. Decreasing the perpendicular distance from the axis reduces the moment of a given force.
8. What do you understand by the clockwise and anticlockwise moment of force? When is it taken positive?
8. What do you understand by the clockwise and anticlockwise moment of force? When is it taken positive?
Solution 8.
If the turning effect on the body is anticlockwise, moment of force is called anticlockwise moment and it is taken as positive while if the turning effect on the body is clockwise, moment of force is called clockwise moment and is taken negative.
9. Why is it easier to open a door by applying the force at the free end of it?
9. Why is it easier to open a door by applying the force at the free end of it?
Solution 9.
It is easier to open a door by applying the force at the free end of it because larger the perpendicular distance , less is the force needed to turn the body.
10. The stone of hand flour grinder is provided with a handle near its rim. Give a reason.
10. The stone of hand flour grinder is provided with a handle near its rim. Give a reason.
Solution 10.
The stone of hand flour grinder is provided with a handle near its rim so that it can be rotated easily about the iron pivot at its centre by a small force applied at the handle.
11. It is easier to turn the steering wheel of a large diameter than that of a small diameter. Give reason.
11. It is easier to turn the steering wheel of a large diameter than that of a small diameter. Give reason.
Solution 11.
It is easier to turn the steering wheel of a large diameter than that of a small diameter because less force is applied on steering of large diameter which is at a large distance from the centre of rim.
12. A spanner (or wrench) has a long handle. Why?
12. A spanner (or wrench) has a long handle. Why?
Solution 12.
A spanner (or wrench) has a long handle to produce larger turning moment so that nut can easily be turned with a less force.
13. A, B and C are the three forces each of magnitude 4N acting in the plane of paper as shown in Figure. The point O lies in the same plane.
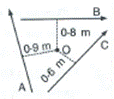
Which force has the least moment about O? Give a reason.
Which force has the greatest moment about O? Give a reason.
Name the forces producing (a) clockwise and (b) anticlockwise moments.
What is the resultant torque about the point O?
13. A, B and C are the three forces each of magnitude 4N acting in the plane of paper as shown in Figure. The point O lies in the same plane.

Which force has the least moment about O? Give a reason.
Which force has the greatest moment about O? Give a reason.
Name the forces producing (a) clockwise and (b) anticlockwise moments.
What is the resultant torque about the point O?
Solution 13.
14. The adjacent diagram shows a heavy roller, with its axle at O, which is to be raised on a pavement XY. If there is friction between the roller and pavement, show by an arrow on the diagram the point of application and the direction of force to be applied.
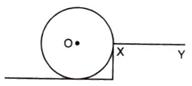
Solution 14.
15. A body is acted upon by two forces each of magnitude F, but in opposite directions. State the effect of the forces if
(a)both forces act at the same point of the body.
(b)the two forces act at two different points of the body at a separation r.
Solution 15.
(a) Resultant force acting on the body = F-F=0 moment of forces = 0 i.e., no motion of the body
(b) The forces tend to rotate the body about the mid-point between two forces, Moment of forces= Fr
16. Draw a neat labelled diagram to show the direction of two forces acting on a body to produce rotation in it. Also mark the point O about which the rotation takes place.
Solution 16.
At A and B, two equal and opposite forces each of magnitude F are applied. The two forces rotate the bar in anticlockwise direction.
17. What do you understand by the term couple? State its effect. Give two examples in our daily life where couple is applied to turn a body.
17. What do you understand by the term couple? State its effect. Give two examples in our daily life where couple is applied to turn a body.
Solution 17.
Two equal and opposite parallel forces not acting along the same line, form a couple. A couple is always needed to produce the rotation. For example, turning a key in a lock and turning a steering wheel.
18. Define moment of a couple. Write its S.I unit.
18. Define moment of a couple. Write its S.I unit.
Solution 18.
The moment of a couple is equal to the product of the either force and the perpendicular distance between the line of action of both the forces. S.I unit of moment of couple is Nm.
19. Prove that
Moment of couple = Force x Couple arm.
19. Prove that
Moment of couple = Force x Couple arm.
Solution 19.
At A and B, two equal and opposite forces each of magnitude F are applied. The two forces rotate the bar in anticlockwise direction. The perpendicular distance between two forces is AB which is called the couple arm.
Moment of force F at the end A
= F x OA(anticlockwise)
Moment of force F at the end B
= F x OB(anticlockwise)
Total moment of couple =F x OA + F x OB
= F x (OA +OB)= F x AB
= F x d(anticlockwise)
=Either force x perpendicular distance between the two forces (or couple arm)
Thus, Moment of couple = Force x Couple arm
20. What do you mean by equilibrium of a body?
20. What do you mean by equilibrium of a body?
Solution 20.
When a number of forces acting on a body produce no change in its state of rest or of motion, the body is said to be in equilibrium.
21. State the condition when a body is in (i) static, (ii) dynamic, equilibrium. Give one example each of static and dynamic equilibrium.
21. State the condition when a body is in (i) static, (ii) dynamic, equilibrium. Give one example each of static and dynamic equilibrium.
Solution 21.
(i) When a body remains in the state of rest under the influence of the applied forces, the body is in static equilibrium. For example a book lying on a table is in static equilibrium.
(ii) When a body remains in the same state of motion (translational or rotational), under the influence of the applied forces, the body is said to be in dynamic equilibrium. For example, a rain drop reaches the earth with a constant velocity is in dynamic equilibrium.
22. State two conditions for a body, acted upon by several forces to be in equilibrium.
22. State two conditions for a body, acted upon by several forces to be in equilibrium.
Solution 22.
For a body to be in equilibrium:
- The resultant of all the forces acting on the body should be equal to zero.
- The resultant moment of all the forces acting on the body about the point of rotation should be zero.
23. State the principle of moments. Name one device based on it.
Solution 23.
According to the principle of moments, if the algebraic sum of moments of all the forces acting on the body about the axis of rotation is zero, the body is in equilibrium. A physical balance (or beam balance) works on the principle of moments.
24. Describe a simple experiment to verify the principle of moments, if you are supplied with a metre rule, a fulcrum and two springs with slotted weights.
24. Describe a simple experiment to verify the principle of moments, if you are supplied with a metre rule, a fulcrum and two springs with slotted weights.
Solution 24.
1(MCQ).The moment of a force about a given axis depends:
(a) Only on the magnitude of force
(b) Only on the perpendicular distance of force from the axis
(c) Neither on the force nor on the perpendicular distance of force from the axis
(d) Both on the force and its perpendicular distance from the axis.
Solution 1 (MCQ).
The moment of a force about a given axis depends on both on the force and its perpendicular distance from the axis.
Hint: Moment of force = Force x Perpendicular distance
2(MCQ).A body is acted upon by two unequal forces in opposite directions, but not in same line. The effect is that
(a) The body will have only the rotational motion
(b) The body will have only the translational motion
(c) The body will have neither the rotational motion nor the translational motion
(d) The body will have rotational as well as translational motion.
2(MCQ).A body is acted upon by two unequal forces in opposite directions, but not in same line. The effect is that
(a) The body will have only the rotational motion
(b) The body will have only the translational motion
(c) The body will have neither the rotational motion nor the translational motion
(d) The body will have rotational as well as translational motion.
Solution 2 (MCQ).
The body will have rotational as well as translational motion.
Numericals
1. The moment of a force of 10N about a fixed point O is 5Nm. Calculate the distance of the point O from the line of action of the force.
Solution 1.
Moment of force= force x perpendicular distance of force from point O
Moment of force= F x r
5Nm= 10 x r
R= 5/10 =0.5 m
2. A nut is opened by a wrench of length 10cm. If the least force required is 5.0N, find the moment of force needed to turn the nut.
Solution 2.
Length, r=10cm =0.1m
F= 5N
Moment of force= F x r= 5 x 0.1 = 0.5 Nm
3. A wheel of diameter 2m is shown with axle at O. A force F = 2N is applied at B in the direction shown in figure. Calculate the moment of force about (i) the centre O, and (ii) the point A.
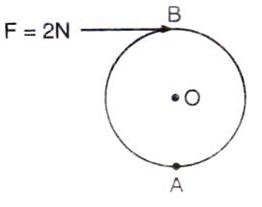
3. A wheel of diameter 2m is shown with axle at O. A force F = 2N is applied at B in the direction shown in figure. Calculate the moment of force about (i) the centre O, and (ii) the point A.

Solution 3.
Given , F= 2N
Diameter=2m
Perpendicular distance between B and O =1m
(i)Moment of force at point O
= F x r
= 2 x 1=2Nm (clockwise)
(ii)Moment of force at point A= F x r
= 2 x 2=4Nm (clockwise)
4. The diagram shows two forces F1= 5N and F2 = 3N acting at points A and B of a rod pivoted at a point O, such that OA = 2m and OB = 4m
Calculate:
(i) the moment of force F1 about O.
(ii) the moment of force F2 about O.
(iii) total moment of the two forces about O.
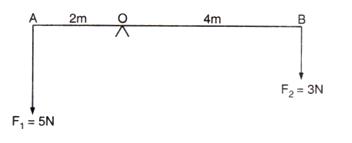
4. The diagram shows two forces F1= 5N and F2 = 3N acting at points A and B of a rod pivoted at a point O, such that OA = 2m and OB = 4m
Calculate:
(i) the moment of force F1 about O.
(ii) the moment of force F2 about O.
(iii) total moment of the two forces about O.

Solution 4.
Given AO=2m and OB=4m
(i) Moment of force F1(=5N) at A about the point O
=F1 x OA
=5 x 2= 10Nm (anticlockwise)
(ii) Moment of force F2(=3N) at B about the point O
= F2 x OB
=3 x 4=12 Nm(clockwise)
(iii) Total moment of forces about the mid-point O
= 12- 10=2Nm(clockwise)
5. Two forces each of magnitude 10N act vertically upwards and downwards respectively at the two ends A and B of a uniform rod of length 4m which is pivoted at its mid-point O as shown. Determine the magnitude of resultant moment of forces about the pivot O.
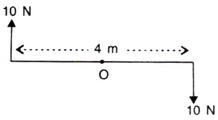
5. Two forces each of magnitude 10N act vertically upwards and downwards respectively at the two ends A and B of a uniform rod of length 4m which is pivoted at its mid-point O as shown. Determine the magnitude of resultant moment of forces about the pivot O.

Solution 5.
Given, AB=4m hence, OA=2m and OB =2m
Moment of force F(=10N) at A about the point O
= F x OA= 10 x 2= 20Nm (clockwise)
Moment of force F (=10N) at point B about the point O
= F x OB= 10 x 2 =20Nm (clockwise)
Total moment of forces about the mid-point O=
=20 +20= 40Nm(clockwise)
6. Figure shows two forces each of magnitude 10N acting at the points A and B at a separation of 50 cm, in opposite directions. Calculate the resultant moment of the two forces about the point (i) A, (ii) B and (iii) O, situated exactly at the middle of the two forces.
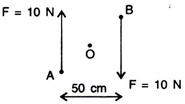
6. Figure shows two forces each of magnitude 10N acting at the points A and B at a separation of 50 cm, in opposite directions. Calculate the resultant moment of the two forces about the point (i) A, (ii) B and (iii) O, situated exactly at the middle of the two forces.

Solution 6.
(i) Perpendicular distance of point A from the force F=10 N at B is 0.5m , while it is zero from the force F=10N at A
Hence, moment of force about A is
= 10 N x 0.5m=5Nm(clockwise)
(ii) Perpendicular distance of point B from the force F=10 N at A is 0.5m, while it is zero from the force F=10N at B
Hence, moment of force about B is
= 10 N x 0.5m=5Nm(clockwise)
(iii) Perpendicular distance of point O from either of the forces F=10N is 0.25 m
Moment of force F(=10N) at A about O= 10N x 0.25m
=2.5Nm(clockwise)
And moment of force F(=10N) at B about O
=10N x 0.25m=2.5Nm(clockwise)
Hence, total moment of the two forces about O
=0.25 + 0.25=5Nm (clockwise)
7. A steering wheel of diameter 0.5m is rotated anti-clockwise by applying two forces each of magnitude 5N. Draw a diagram to show the application of forces and calculate the moment of forces applied.
7. A steering wheel of diameter 0.5m is rotated anti-clockwise by applying two forces each of magnitude 5N. Draw a diagram to show the application of forces and calculate the moment of forces applied.
Solution 7.
8. A uniform metre rule is pivoted at its mid-point. A weight of 50gf is suspended at one end of it. Where a weight of 100gf should be suspended to keep the rule horizontal?
Solution 8.
Let the 50gf weight produce anticlockwise moment about the middle point of metre rule .i.e, at 50cm.
Let a weight of 100gf produce a clockwise moment about the middle point. Let its distance from the middle be d cm. Then,
according to principle of moments,
Anticlockwise moment = Clockwise moment
50gf x 50 cm=100gf x d
9. A uniform metre rule balances horizontally on a knife edge placed at the 58 cm mark when a weight of 20gf is suspended from one end.
(i)Draw a diagram of the arrangement.
(ii)What is the weight of the rule?
Solution 9.
(i) Weight mg (W) of rule produces an anti-clockwise moment about the knife edge O. In order to balance it, 20gf must be suspended at the end B to produce clockwise moment about the knife edge O.
10. The diagram shows a uniform bar supported at the middle point O. A weight of 40gf is placed at a distance 40cm to the left of the point O. How can you balance the bar with a weight of 80gf?

Solution 10.
Anticlockwise moment= 40gf x 40 cm
Clockwise moment= 80gf x d cm
From the principle of moments,
Anticlockwise moment= Clockwise moment
40gf x 40 cm =80gf x d
11. Figure shows a uniform metre rule placed on a fulcrum at its mid-point O and having a weight 40gf at the 10 cm mark and a weight of 20gf at the 90 cm mark. (i) Is the metre rule in equilibrium? If not, how will the rule turn? (ii) How can the rule be brought in equilibrium by using an additional weight of 40gf?

Solution 11.
(i) Anticlockwise moment= 40gf x (50-10)cm
=40gf x 40cm=1600 gf x cm
Clockwise moment= 20gf x (90- 50) =20gf x 40cm
=800 gf x cm
Anticlockwise moment is not equal to clockwise moment. Hence the metre rule is not in equilibrium and it will turn anticlockwise.
(ii) To balance it, 40gf weight should be kept on right hand side so as to produce a clockwise moment about the middle point. Let its distance from the middle be d cm. Then,
clockwise moment= 20gf x 40cm + 40gf x d cm
From the principle of moments,
Anticlockwise moment= Clockwise moment
40 gf x 40 cm= 20gf x 40 + 40 x d cm
1600-800=40gf x dcm
12. When a boy weighing 20kgf sits at one end of a 4m long see-saw, it gets depressed at its end. How can it be brought to the horizontal position by a man weighing 40kgf.
Solution 12.
From the principle of moments,
Anticlockwise moment= Clockwise moment
20kgf x 2m =40kgf x d
13. A physical balance has its arms of length 60 cm and 40 cm. What weight kept on a pan of longer arm will balance an object of weight 100gf kept on other pan?
Solution 13.
14. The diagram shows a uniform metre rule weighing 100gf, pivoted at its centre O. Two weights 150gf and 250gf hang from the point A and B respectively of the metre rule such that OA = 40 cm and OB = 20 cm. Calculate: (i) the total anticlockwise moment about O, (ii) the total clockwise moment about O, (iii) the difference of anticlockwise and clockwise moment, and (iv) the distance from O where a 100gf weight should be placed to balance the metre rule.

Solution 14.
(i) Total anticlockwise moment about O
= 150gf x 40 cm=6000gf cm
(ii) Total clockwise moment about O,
=250gf x 20 cm= 5000gf cm
(iii) The difference of anticlockwise and clockwise moment= 6000- 5000= 1000gf cm
(iv) From the principle of moments,
Anticlockwise moment= Clockwise moment
To balance it, 100gf weight should be kept on right hand side so as to produce a clockwise moment about the O. Let its distance from the point O be d cm. Then,
150gf x 40 cm=250gf x 20 cm +100gf x d
6000gf cm= 5000gf cm + 100gf x d
1000gf cm =100 gf x d
15. A uniform metre rule of weight 10gf is pivoted at its 0 mark. (i) What moment of force depresses the rule? (ii) How can it be made horizontal by applying a least force?
Solution 15.
Solution 16.
17. A uniform metre rule of mass 100g is balanced on a fulcrum at mark 40cm by suspending an unknown mass m at the mark 20cm. (i) find the value of m. (ii) To which side the rule will tilt if the mass m is moved to the mark 10cm? (iii) What is the resultant moment now? (iv) How can it be balanced by another mass 50 g?
Solution 17.
(i) From the principle of moments,
Clockwise moment= Anticlockwise moment
100g x (50-40) cm= mx(40-20) cm
100g x 10 cm = m x 20 cm = m =50 g
(ii) The rule will tilt on the side of mass m (anticlockwise), if the mass m is moved to the mark 10cm.
(iii) Anticlockwise moment if mass m is moved to the mark 10 cm= 50g x (40-10)cm =50 x 30=1500g cm
Clockwise moment=100g x (50-40) cm= 1000g cm
Resultant moment= 1500g cm -1000g cm= 500g cm (anticlockwise)
(iv) From the principle of moments,
Clockwise moment= Anticlockwise moment
To balance it, 50g weight should be kept on right hand side so as to produce a clockwise moment .Let its distance from fulcrum be d cm. Then,
100g x (50-40) cm + 50g x d =50g x (40-10)cm
1000g cm + 50g x d =1500 g cm
50 g x d= 500g cm
So, d =10 cm
By suspending the mass 50g at the mark 50 cm, it can be balanced.
Exercise 1(B)
1. Define the term 'centre of gravity of a body'.
Solution 1.
Centre of gravity is the point about which the algebraic sum of moments of weights of particles constituting the body is zero and the entire weight of the body is considered to act at this point.
2. Can the centre of gravity of a body be situated outside its material of the body? Give an example.
2. Can the centre of gravity of a body be situated outside its material of the body? Give an example.
Solution 2.
Yes, the centre of gravity can be situated outside the material of the body. For example, centre of gravity of ring.
3. State factor on which the position of centre of gravity of a body depend? Explain your answer with an example.
3. State factor on which the position of centre of gravity of a body depend? Explain your answer with an example.
Solution 3.
The position of centre of gravity of a body of given mass depends on its shape i.e., on the distribution of mass in it. For example: the centre of gravity of a uniform wire is at its mid-point. But if this wire is bent into the form of a circle, its centre of gravity will then be at the centre of circle.
4. What is the position of centre of gravity of a :
(a) rectangular lamina
(b) cylinder ?
4. What is the position of centre of gravity of a :
(a) rectangular lamina
(b) cylinder ?
Solution 4.
The position of centre of gravity of a
(a) rectangular lamina is at the point of intersection of its diagonals.
(b) cylinder is at the mid point on the axis of cylinder.
5. At which point is the centre of gravity of situated in:
(a) A triangular lamina and
(b) A circular lamina?
5. At which point is the centre of gravity of situated in:
(a) A triangular lamina and
(b) A circular lamina?
Solution 5.
(a) Centre of gravity of a triangular lamina is situated at the point of intersection of its medians.
(b) Centre of gravity of a circular lamina is situated at the centre of circular lamina.
6. Where is the centre of gravity of a uniform ring situated?
6. Where is the centre of gravity of a uniform ring situated?
Solution 6.
Centre of gravity of a uniform ring is situated at the centre of ring.
7. A square card board is suspended by passing a pin through a narrow hole at its one corner. Draw a diagram to show its rest position. In the diagram mark the point of suspension by the letter S and centre of gravity by the letter G.
7. A square card board is suspended by passing a pin through a narrow hole at its one corner. Draw a diagram to show its rest position. In the diagram mark the point of suspension by the letter S and centre of gravity by the letter G.
Solution 7.
8. Explain how will you determine experimentally the position of centre of gravity for a triangular lamina (or a triangular piece of card board).
Solution 8.
Take a triangular lamina. Make three fine holes at a, b, c near the edge of triangular lamina. Now suspend the given lamina along with a plumb line from hole ‘a’. Check that the lamina is free to oscillate about the point of suspension. When lamina has come to rest, draw straight line ad along the plumb line. Repeat the experiment by suspending the lamina through hole ‘b’ and then through hole ‘c’ for which we get straight lines be and cf respectively. It is noticed that the lines ad, be and cf intersect each other at a common point G which is the position of centre of gravity of triangular lamina i.e. the point of intersection of medians.
9. State whether the following statement is true or false.
(i) 'The position of centre of gravity of a body remains unchanged even when the body is deformed'.
(ii) 'The centre of gravity of a freely suspended body always lies vertically below the point of suspension'.
Solution 9.
(i) False. The position of centre of gravity of a body of given mass depends on its shape i.e., on the distribution of mass in it.
(ii) True.
10. A uniform flat circular rim is balanced on a sharp vertical nail by supporting it at point A, as shown in Fig. 1.42. Mark the position of centre of gravity of the rim in the diagram by the letter G.
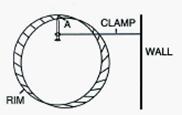
10. A uniform flat circular rim is balanced on a sharp vertical nail by supporting it at point A, as shown in Fig. 1.42. Mark the position of centre of gravity of the rim in the diagram by the letter G.

Solution 10.
11. Figure shows three pieces of card board of uniform thickness cut into three different shapes. On each diagram draw two lines to indicate the position of centre of gravity G.
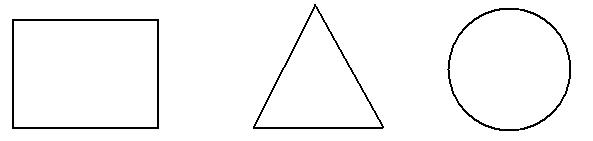
Solution 11.
Solution 1 (MCQ).
At its geometrical centre
Exercise 1(C)
1. Explain the meaning of uniform circular motion. Why is such motion said to be accelerated?
Solution 1.
When a particle moves with a constant speed in a circular path, its motion is said to be the uniform circular motion. For example : Revolution of earth around sun is an example of uniform circular motion.
2. Draw a neat labelled diagram for a particle moving in a circular path with a constant speed. In your diagram show the direction of velocity at any instant.
2. Draw a neat labelled diagram for a particle moving in a circular path with a constant speed. In your diagram show the direction of velocity at any instant.
Solution 2.
Solution 3.
Yes, uniform circular motion has an accelerated motion with a constant speed.
4. Give one example of motion in which speed remains uniform, but the velocity changes.
4. Give one example of motion in which speed remains uniform, but the velocity changes.
Solution 4.
Motion of a cyclist on a circular track is an example of motion in which speed remains uniform, but the velocity changes.
5. A uniform circular motion is an accelerated motion. Explain it. State whether the acceleration is uniform or variable? Name the force responsible to cause this acceleration. What is the direction of force at any instant? Draw diagram in support of your answer.
5. A uniform circular motion is an accelerated motion. Explain it. State whether the acceleration is uniform or variable? Name the force responsible to cause this acceleration. What is the direction of force at any instant? Draw diagram in support of your answer.
Solution 5.
When the object moves in a circular path with uniform speed, it means that its magnitude of velocity does not change, only its direction changes continuously. Hence, it is considered as uniformly accelerated motion.
6. Differentiate between a uniform linear motion and a uniform circular motion.
6. Differentiate between a uniform linear motion and a uniform circular motion.
Solution 6.
Solution 7.
Centripetal force is required for circular motion. It is always directed towards the centre of circle.
8. What is centripetal force?
8. What is centripetal force?
Solution 8.
Force acting on a body which is in circular motion is called centripetal force. It acts towards the centre of circular path.
9. Explain the motion of a planet around the sun in a circular path.
9. Explain the motion of a planet around the sun in a circular path.
Solution 9.
A planet moves around the sun in a nearly circular path for which the gravitational force of attraction on the planet by the sun provides the necessary centripetal force required for circular motion.
10.(a) How does a centripetal force differ from a centrifugal force with reference to the direction in which they act?
(b) Is centrifugal force the force of reaction of centripetal force?
(c) Compare the magnitudes of centripetal and centrifugal force.
10.(a) How does a centripetal force differ from a centrifugal force with reference to the direction in which they act?
(b) Is centrifugal force the force of reaction of centripetal force?
(c) Compare the magnitudes of centripetal and centrifugal force.
Solution 10.
(a) They act in opposite directions.
(b) No, centrifugal force is not the force of reaction of centripetal force.
11. Is centrifugal force a real force?
11. Is centrifugal force a real force?
Solution 11.
No, centrifugal force is a fictitious force.
12. A small pebble tied at one end of a string is placed near the periphery of a circular disc, at the center of which the other end of the string is tied to a peg. The disc is rotating about an axis passing through its centre.
(a)What will be your observation when you are standing outside the disc? Explain.
(b)What will be your observation when you are standing at the centre of the disc. Explain.
12. A small pebble tied at one end of a string is placed near the periphery of a circular disc, at the center of which the other end of the string is tied to a peg. The disc is rotating about an axis passing through its centre.
(a)What will be your observation when you are standing outside the disc? Explain.
(b)What will be your observation when you are standing at the centre of the disc. Explain.
Solution 12.
a. On standing outside the disc, we find that the pebble is moving on a circular path. On standing at the centre of the disc, we find that the pebble is stationary placed just in front of us.
13. A piece of stone tied at the end of a thread is whirled in a horizontal circle with uniform speed with the help of hand. Answer the following questions.
a. Is the velocity of stone uniform or variable?
b. Is the acceleration of stone uniform or variable?
c. What is the direction of acceleration of stone at any instant?
d. What force does provide the centripetal force required for circular motion?
e. Name the force and its direction which acts on the hand.
13. A piece of stone tied at the end of a thread is whirled in a horizontal circle with uniform speed with the help of hand. Answer the following questions.
a. Is the velocity of stone uniform or variable?
b. Is the acceleration of stone uniform or variable?
c. What is the direction of acceleration of stone at any instant?
d. What force does provide the centripetal force required for circular motion?
e. Name the force and its direction which acts on the hand.
Solution 13.
Force of tension in the thread provides the centripetal force.
14. State whether the following statements are true or false by writing T/F against them.
The earth moves around the sun with a uniform velocity.
The motion of the moon around the earth in circular path is an accelerated motion.
A uniform linear motion is unaccelerated, while a uniform circular motion is an accelerated motion.
In a uniform circular motion, the speed continuously changes because the direction of the motion changes.
14. State whether the following statements are true or false by writing T/F against them.
The earth moves around the sun with a uniform velocity.
The motion of the moon around the earth in circular path is an accelerated motion.
A uniform linear motion is unaccelerated, while a uniform circular motion is an accelerated motion.
In a uniform circular motion, the speed continuously changes because the direction of the motion changes.
Solution 14.
(a) False
(b) True
(c) True
(d) False
1(MCQ). Which of the following quantity remains constant in uniform circular motion:
(a) Velocity
(b) Speed
(c) Acceleration
(d) Both velocity and speed
1(MCQ). Which of the following quantity remains constant in uniform circular motion:
(a) Velocity
(b) Speed
(c) Acceleration
(d) Both velocity and speed
Solution 1 (MCQ).
Speed
Hint: Speed is scalar but velocity and acceleration are vector quantities. So, speed remains constant but velocity and acceleration change with the change in direction, and in circular motion the direction of motion changes at every point.





















