Selina Concise Mathematics Class 9 ICSE Solutions Chapter 10 - Isosceles Triangles

Selina Concise Mathematics Class 9 ICSE Solutions Isosceles Triangles

Selina ICSE Solutions for Class 9 Maths Chapter 10 Isosceles Triangles

Exercise 10(A)

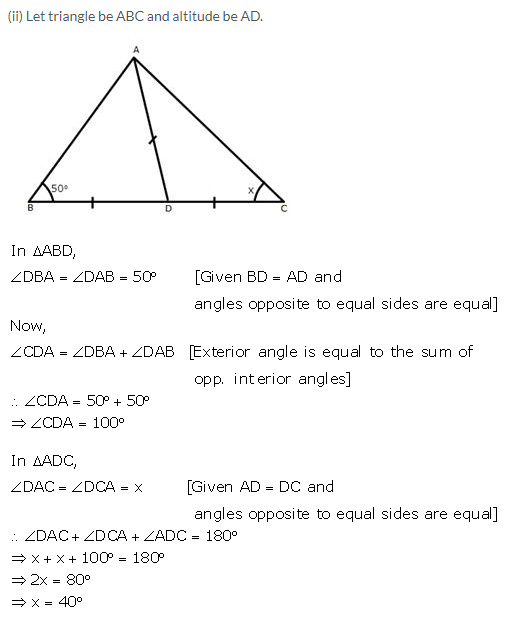
1. In the figure alongside,



AB = AC
A = 48^o and

ACD = 18^o.

Show that BC = CD.

Solution 1:

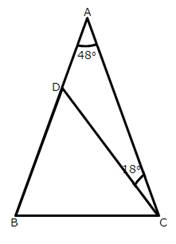
2. Calculate:

(i) ADC

(ii) ABC

(iii) BAC

Solution 2:

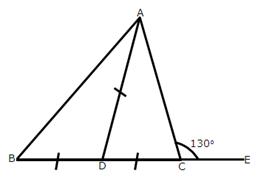
3. In the following figure, AB = AC; BC = CD and DE is parallel to BC. Calculate:

(i) CDE

(ii) 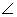DCE

Solution 3:

4. Calculate x:

(i)


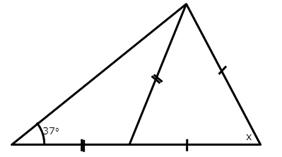
(ii)


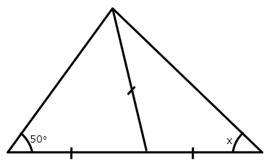
Solution 4:

5. In the figure, given below, AB = AC. Prove that: BOC = ACD.




Solution 5:

6. In the figure given below, LM = LN; angle PLN = 110o. Calculate:

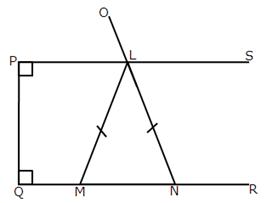
(i) LMN

(ii) MLN

Solution 6:

7. An isosceles triangle ABC has AC = BC. CD bisects AB at D and CAB = 55^o.

Find: (i) 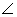DCB (ii) 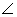CBD.

Solution 7:

8. Find x:



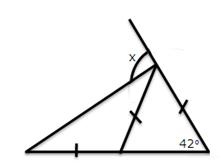
Solution 8:

9. In the triangle ABC, BD bisects angle B and is perpendicular to AC. If the lengths of the sides of the triangle are expressed in terms of x and y as shown, find the values of x and y.



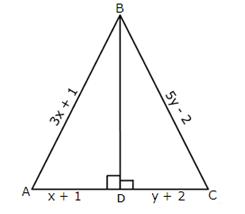
Solution 9:

10. In the given figure; AE // BD, AC // ED and AB = AC. Find  a, b and c.

Solution 10:

11. In the following figure; AC = CD, AD = BD and   C = 58^o.



Find angle CAB.

Solution 11:

12. In the figure of q. no. 11 given above, if AC = AD = CD = BD; find angle ABC.



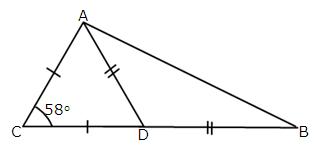
Solution 12:

13. In triangle ABC; AB = AC and A : B = 8 : 5; find angle A.

Solution 13:

14. In triangle ABC; A = 60^o, C = 40^o, and bisector of angle ABC meets side AC at point P. Show that BP = CP.

Solution 14:

15. In triangle ABC; angle ABC = 90o and P is a point on AC such that   PBC = PCB  Show that: PA = PB.

Solution 15:

16. ABC is an equilateral triangle. Its side BC is produced upto point E such that C is mid-point of BE. Calculate the measure of angles ACE and AEC.

Solution 16:

17. In triangle ABC, D is a point in AB such that AC = CD = DB. If ∠B = 28°, find the angle ACD.

Solution 17:

18. In the given figure, AD = AB = AC, BD is parallel to CA and angle ACB = 65°. Find angle DAC.



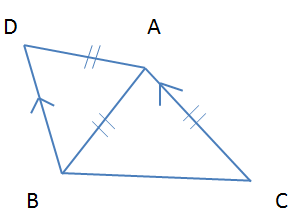
Solution 18:

Exercise 10(B)

1. If the equal sides of an isosceles triangle are produced, prove that the exterior angles so formed are obtuse and equal.

Solution 1:

2. In the given figure, AB = AC. Prove that:



(i) DP = DQ

(ii) AP = AQ

(iii) AD bisects angle A

Solution 2:

3. In triangle ABC, AB = AC; BE AC and CF AB. Prove that:

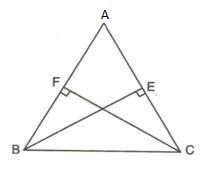
(i) BE = CF

(ii) AF = AE

Solution 3:

4. In isosceles triangle ABC, AB = AC. The side BA is produced to D such that BA = AD. Prove that: BCD = 90^o

Solution 4:

5. (i) In triangle ABC, AB = AC and = 36°. If the internal bisector of meets AB at point D, prove that AD = BC.

(ii) If the bisector of an angle of a triangle bisects the opposite side, prove that the triangle is isosceles.

Solution 5:

6. Prove that the bisectors of the base angles of an isosceles triangle are equal.

Solution 6:

7. In the given figure, AB = AC and DBC = ECB = 90^o 



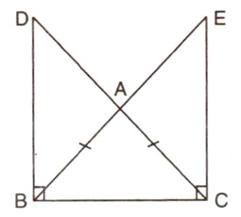
Prove that:

(i) BD = CE

(ii) AD = AE

Solution 7:

8. ABC and DBC are two isosceles triangles on the same side of BC. Prove that:

(i) DA (or AD) produced bisects BC at right angle.
(ii) BDA = CDA.

Solution 8:

9. The bisectors of the equal angles B and C of an isosceles triangle ABC meet at O. Prove that AO bisects angle A.

Solution 9:

10. Prove that the medians corresponding to equal sides of an isosceles triangle are equal.

Solution 10:

11. Use the given figure to prove that, AB = AC.




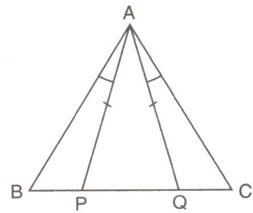
Solution 11:

12. In the given figure; AE bisects exterior angle CAD and AE is parallel to BC.

Prove that: AB = AC.




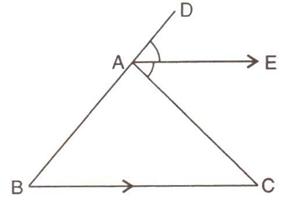
Solution 12:

13. In an equilateral triangle ABC; points P, Q and R are taken on the sides AB, BC and CA respectively such that AP = BQ = CR. Prove that triangle PQR is equilateral.

Solution 13:

14. In triangle ABC, altitudes BE and CF are equal. Prove that the triangle is isosceles.

Solution 14:

15. Through any point in the bisector of angle, a straight line is drawn parallel to either arm of the angle. Prove that the triangle so formed is isosceles.

Solution 15:

16. In triangle ABC; AB = AC. P, Q and R are mid-points of sides AB, AC and BC respectively. Prove that:

(i) PR = QR(ii) BQ = CP

Solution 16:

17. From the following figure, prove that:

(i) ACD = CBE

(ii) AD = CE

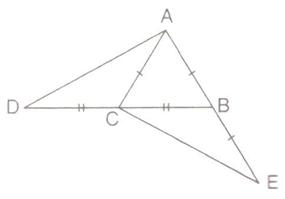
Solution 17:

18. Equal sides AB and AC of an isosceles triangle ABC are produced. The bisectors of the exterior angle so formed meet at D. Prove that AD bisects angle A.

Solution 18:

 AB is produced to E and AC is produced to F. BD is bisector of angle CBE and CD is bisector of angle BCF. BD and CD meet at D.

19. ABC is a triangle. The bisector of the angle BCA meets AB in X. A point Y lies on CX such that AX = AY.

Prove that  CAY = ABC.

Solution 19:

20. In the following figure; IA and IB are bisectors of angles CAB and CBA respectively. CP is parallel to IA and CQ is parallel to IB.



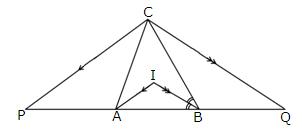
Prove that:

PQ = The perimeter of the  ABC.

Solution 20:

21. Sides AB and AC of a triangle ABC are equal. BC is produced through C upto a point D such that AC = CD. D and A are joined and produced upto point E. If angle BAE = 108o; find angle ADB.

Solution 21:

22. The given figure shows an equilateral triangle ABC with each side 15 cm. Also, DE//BC, DF//AC and EG//AB.
If DE + DF + EG = 20 cm, find FG.




Solution 22:

23. If all the three altitudes of a triangle are equal, the triangle is equilateral. Prove it.

Solution 23:

24. In a ABC, the internal bisector of angle A meets opposite side BC at point D. Through vertex C, line CE is drawn parallel to DA which meets BA produced at point E. Show that ACE is isosceles.

Solution 24:

25. In triangle ABC, bisector of angle BAC meets opposite side BC at point D. If BD = CD, prove that Selina Solutions Icse Class 9 Mathematics Chapter - Isosceles TriangleABC is isosceles.

Solution 25:

26. In ABC, D is point on BC such that AB = AD = BD = DC. Show that:

ADC: C = 4: 1.

Solution 26:

27. Using the information given in each of the following figures, find the values of a and b. 


 

 [Given: CE = AC] 

  

Solution 27:

Selina Concise Mathematics Class 9 ICSE Solutions Chapter 10 - Isosceles Triangles