Selina Concise Mathematics Class 9 ICSE Solutions Chapter 6 - Simultaneous (Linear) Equations (Including Problems)

Selina Concise Mathematics Class 9 ICSE Solutions Simultaneous (Linear) Equations (Including Problems)

Selina ICSE Solutions for Class 9 Maths Chapter 6 Simultaneous (Linear) Equations (Including Problems)

Exercise 6(A)


1.Solve: 3(2x + 1) - 2x+2 + 5 = 0.Solve the pairs of linear (simultaneous) equations by the method of elimination by substitution:

8x + 5y = 9

3x + 2y = 4

Solution 1:

2.Solve the pairs of linear (simultaneous) equations by the method of elimination by substitution:

2x - 3y = 7

5x + y= 9

Solution 2:

3.Solve the pairs of linear (simultaneous) equations by the method of elimination by substitution:

2x + 3y = 8

2x = 2 + 3y

Solution 3:

4.Solve the following pairs of linear (simultaneous) equations by the method of elimination by substitution:
0.2x + 0.1y = 25
2(x - 2) - 1.6y = 116

Solution 4:

5.Solve the pairs of linear (simultaneous) equations by the method of elimination by substitution:

6x = 7y + 7

7y - x = 8

Solution 5:

6.Solve the pairs of linear (simultaneous) equations by the method of elimination by substitution:

y = 4x - 7

16x - 5y = 25

Solution 6:

7.Solve the pairs of linear (simultaneous) equations by the method of elimination by substitution:

2x + 7y = 39

3x + 5y = 31

Solution 7:

8.Solve the following pairs of linear (simultaneous) equations by the method of elimination by substitution:



1.5x + 0.1y = 6.2



3x - 0.4y = 11.2

Solution 8:

9.

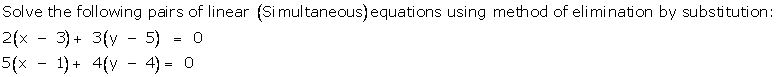Solution 9:

10.


Solution 10:

Exercise 6(B)

1.For solving each pair of equation, in this exercise use the method of elimination by equating coefficients:

13 + 2y = 9x

3y = 7x

Solution 1:

2.For solving each pair of equation, in this exercise use the method of elimination by equating coefficients:

3x - y = 23

Solution 2:

3.For solving each pair of equation, in this exercise use the method of elimination by equating coefficients:

Solution 3:

4.For solving each pair of equation, in this exercise use the method of elimination by equating coefficients:


Solution 4:

5.For solving each pair of equation, in this exercise use the method of elimination by equating coefficients:

y = 2x - 6

y = 0

Solution 5:

6.For solving each pair of equation, in this exercise use the method of elimination by equating coefficients:



Solution 6:

7.For solving each pair of equation, in this exercise use the method of elimination by equating coefficients:

3 - (x - 5) = y + 2

2 (x + y) = 4 - 3y

Solution 7:

8.For solving each pair of equation, in this exercise use the method of elimination by equating coefficients:

2x - 3y - 3 = 0

Solution 8:

9.For solving each pair of equation, in this exercise use the method of elimination by equating coefficients:

13x+ 11y = 70

11x + 13y = 74

Solution 9:

10.For solving each pair of equation, in this exercise use the method of elimination by equating coefficients:

41x + 53y = 135

53x + 41y = 147

Solution 10:

11.If 2x + y = 23 and 4x - y = 19; find the values of x - 3y and 5y - 2x.

Solution 11:

12.If 10y = 7x - 4 and 12x + 18y = 1; find the values of 4x + 6y and 8y - x.

Solution 12:

13.Solve for x and y:

Solution 13:

14.Find the value of m, if x = 2, y = 1 is a solution of the equation 2x + 3y = m.

Solution 14:

15.10% of x + 20% of y = 24

 3x - y = 20

Solution 15:

16.The value of expression mx - ny is 3 when x = 5 and y = 6. And its value is 8 when x = 6 and y = 5. Find the values of m and n.

Solution 16:

17.Solve:

11(x - 5) + 10(y - 2) + 54 = 0

7(2x - 1) + 9(3y - 1) = 25

Solution 17:

18.



Solution 18:

19.
 

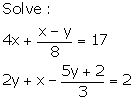
Solution 19:

Exercise 6(C)

1.Solve, using cross-multiplication :

4x + 3y = 17

3x - 4y + 6 = 0

Solution 1:

2.Solve, using cross-multiplication :

3x + 4y = 11

2x + 3y = 8

Solution 2:

3.Solve, using cross-multiplication :

6x + 7y - 11 = 0

5x + 2y = 13

Solution 3:

4.Solve, using cross-multiplication :

5x + 4y + 14 = 0

3x = -10 - 4y

Solution 4:

5.Solve, using cross-multiplication :

x - y + 2 = 0

7x + 9y = 130

Solution 5:

6.Solve, using cross-multiplication :

4x - y = 5

5y - 4x = 7

Solution 6:

7.Solve, using cross-multiplication :

4x - 3y = 0

2x + 3y = 18

Solution 7:

8.Solve, using cross-multiplication :

8x + 5y = 9

3x + 2y = 4

Solution 8:

9.Solve, using cross-multiplication :

4x - 3y - 11 = 0

6x + 7y - 5 = 0

Solution 9:

10.Solve, using cross-multiplication :

4x + 6y = 15

3x - 4y = 7

Solution 10:

Exercise 6(D)

1.


Solution 1:

2.Solve the pairs of equations:


Solution 2:

3.

Solution 3:

4.

Solution 4:

5.Solve: 

 

Hence, find 'a' if y = ax + 3.

Solution 5:

6.Solve:

Solution 6:

7.Solve:

(i) x + y = 2xy

x - y = 6xy

(ii) x+ y = 7xy

2x - 3y = -xy

Solution 7:

8.

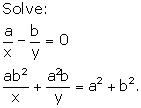
Solution 8:

9.

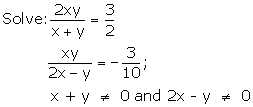
Solution 9:

 

10.

 

Solution 10:

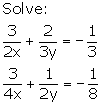
Exercise 6(E)
1.The ratio of two numbers is . If 2 is subtracted from the first and 8 from the second, the ratio becomes the reciprocal of the original ratio. Find the numbers.

Solution 1:

2.Two numbers are in the ratio 4 : 7. If thrice the larger be added to twice the smaller, the sum is 59. Find the numbers.

Solution 2:

3.When the greater of the two numbers increased by 1 divides the sum of the numbers, the result is. When the difference of these numbers is divided by the smaller, the result. Find the numbers.

Solution 3:

4.Two numbers are in the ratio 4:5. If 30 is subtracted from each of the numbers, the ratio becomes 1:2. Find the numbers.

Solution 4:

5.If the numerator of a fraction is increased by 2 and denominator is decreased by 1, it becomes 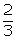. If the numerator is increased by 1 and denominator is increased by 2, it becomes 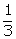. Find the fraction.

Solution 5:

6.The sum of the numerator and the denominator of a fraction is equal to 7. Four times the numerator is 8 less than 5 times the denominator. Find the fraction.

Solution 6:

7.


Solution 7:

8.


Solution 8:

9.The sum of the digits of a two digit number is 7. If the digits are reversed, the new number decreased by 2, equals twice the original number. Find the number.

Solution 9:

10.The ten’s digit of a two digit number is three times the unit digit. The sum of the number and the unit digit is 32. Find the number.

Solution 10:

11.A two-digit number is such that the ten’s digit exceeds twice the unit’s digit by 2 and the number obtained by inter-changing the digits is 5 more than the the sum of the digits. Find the two digit number.

Solution 11:

12.Four times a certain two digit number is seven times the number obtained on interchanging its digits. If the difference between the digits is 4; find the number.

Solution 12:

13.The sum of two digit number and the number obtained by interchanging the digits of the number is 121. If the digits of the number differ by 3, find the number.

Solution 13:

14.A two digit number is obtained by multiplying the sum of the digits by 8. Also, it is obtained by multiplying the difference of the digits by 14 and adding 2. Find the number.

Solution 14:

Exercise 6(F)

1.Five years ago, A's age was four times the age of B. Five years hence, A’s age will be twice the age of B. Find their preset ages.

Solution 1:

2.A is 20 years older than B. 5 years ago, A was 3 times as old as B. Find their present ages.

Solution 2:

3.Four years ago, a mother was four times as old as her daughter. Six years later, the mother will be two and a half times as old as her daughter at that time. Find the present ages of mother and her daughter.

Solution 3:

4.The age of a man is twice the sum of the ages of his two children. After 20 years, his age will be equal to the sum of the ages of his children at that time. Find the present age of the man.

Solution 4:

5.The annual incomes of A and B are in the ratio 3 : 4 and their annual expenditure are in the ratio 5 : 7. If each Rs. 5000; find their annual incomes.

Solution 5:

6.In an examination, the ratio of passes to failures was 4 : 1. Had 30 less appeared and 20 less passed, the ratio of passes to failures would have been 5 : 1. Find the number of students who appeared for the examination.

Solution 6:

7.A and B both the have some pencils. If A gives 10 pencils to B, then B will have twice as many as A. And if B gives 10 pencils to A, then they will have the same number of pencils. How many pencils does each have?

Solution 7:

8.1250 persons went to sea a circus-show. Each adult paid Rs. 75 and each child paid Rs. 25 for the admission ticket. Find the number of adults and number of children, if the total collection from them amounts to Rs. 61,250.

Solution 8:

9.Two articles A and B are sold for Rs. 1,167 making 5% profit on A and 7% profiton A and 7% profit on B. IF the two articles are sold for Rs. 1,165, a profit of 7% is made on A and a profit of 5% is made on B. Find the cost prices of each article.

Solution 9:

10.Pooja and Ritu can do a piece of work in days. If one day work of Pooja be three fourth of one day work of Ritu’ find in how many days each will do the work alone. 

Solution 10:

Exercise 6(G)

1.Rohit says to Ajay, “Give me hundred, I shall then become twice as rich as you.” Ajay replies, “if you give me ten, I shall be six times as rich as you.” How much does each have originally?

Solution 1:

2.The sum of a two digit number and the number obtained by reversing the order of the digits is 99. Find the number, if the digits differ by 3.

Solution 2:

3.Seven times a two digit number is equal to four times the number obtained by reversing the digits. If the difference between the digits is 3 find the number.

Solution 3:

4.From Delhi station, if we buy 2 tickets for station A and 3 tickets for station B, the total cost is Rs. 77. But if we buy 3 tickets for station A and 5 tickets for station B, the total cost is Rs. 124. What are the fares from Delhi to station A and to station B?

Solution 4:

5.The sum of digit of a two digit number is 11. If the digit at ten's place is increased by 5 and the digit at unit place is decreased by 5, the digits of the number are found to be reversed. Find the original number.

Solution 5:

6.90% acid solution (90% pure acid and 10% water) and 97% acid solution are mixed to obtain 21 litres of 95% acid solution. How many litres of each solution are mixed.

Solution 6:

7.The class XI students of school wanted to give a farewell party to the outgoing students of class XII. They decided to purchase two kinds of sweets, one costing Rs. 250 per kg and other costing Rs. 350 per kg. They estimated that 40 kg of sweets were needed. If the total budget for the sweets was Rs. 11,800; find how much sweets of each kind were bought?



Solution 7:

8.Mr. and Mrs. Abuja weight x kg and y kg respectively. They both take a dieting course, at the end of which Mr. Ahuja loses 5 kg and weights as much as his wife weighed before the course.

Mrs. Ahuja loses 4 kg and weighs 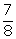th of what her husband weighed before the course. Form two equations in x and y, find their weights before taking the dieting course.

Solution 8:

9.A part of monthly expenses of a family is constants and the remaining vary with the number of members in the family. For a family of 4 person, the total monthly expenses are Rs. 10,400 whereas for a family of 7 persons, the total monthly expenses are Rs. 15,800. Find the constant expenses per month and the monthly expenses of each member of a family.

Solution 9:

10.The taxi charges in a city consist of a fixed charge together with the charge for the distance covered. For a distance of 10 km, the charge paid is Rs. 315 and for a journey of 15 km, the charge paid is Rs. 465. What are the fixed charges and the charge per kilometer? How much does a person have to pay for travelling a distance of 32 km?

Solution 10:

11.A lending library has a fixed charge for the first three days and an additional charge for each day thereafter. Geeta paid Rs. 27 for a book kept for seven days, while Mohit paid Rs. 21 for the book he kept for five days. Find the fixed charges and the charge for each extra day.

Solution 11:

12.The area of a rectangle gets reduced by 9 square units, if its length is reduced by 5 units and breadth is increased by 3 units. However, if the length of this rectangle increases by 3 units and the breadth by 2 units, the area increases by 67 square units. Find the dimensions of the rectangle.

Solution 12:

13.It takes 12 hours to fill a swimming pool using two pipes. If the pipe of larger diameter is used for 4 hours and the pipe of smaller diameter is used for 9 hours, only half of the pool is filled. How long would each pipe take to fill the swimming pool?

Solution 13:

Selina Concise Mathematics Class 9 ICSE Solutions Chapter 6 - Simultaneous (Linear) Equations (Including Problems)