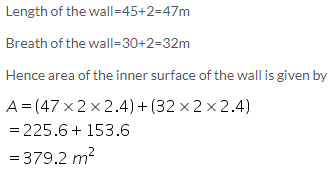Selina Concise Mathematics Class 9 ICSE Maths Solutions Chapter 20 - Area and Perimeter of Plane Figures
Selina Concise Mathematics Class 9 ICSE Solutions Area and Perimeter of Plane Figures
Selina ICSE Solutions for Class 9 Maths Chapter 20 Area and Perimeter of Plane Figures
Exercise 20(A)1.Find the area of a triangle whose sides are 18 cm, 24 cm and 30 cm.
Also, find the length of altitude corresponding to the largest side of the triangle.
Solution 1:
2.The length of the sides of a triangle are in the ratio 3: 4: 5. Find the area of the triangle if its perimeter is 144 cm.
Solution 2:
3.ABC is a triangle in which AB = AC = 4 cm and
A = 90o. Calculate:
(i) The area of 
ABC,
ABC,
(ii) The length of perpendicular from A to BC.
Solution 3:
4.The area of an equilateral triangle is
sq. cm. Find its perimeter.Solution 4:
5.Find the area of an isosceles triangle with perimeter is 36 cm and base is 16 cm.
Solution 5:
Since the perimeter of the isosceles triangle is 36cm and base is 16cm.
6.The base of an isosceles triangle is 24 cm and its area is 192 sq. cm. Find its perimeter.
Solution 6:
7.The given figure shows a right-angled triangle ABC and an equilateral triangle BCD. Find the area of the shaded portion.
Solution 7:
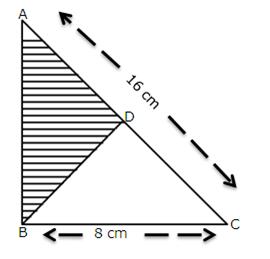
8.Find the area and the perimeter of quadrilateral ABCD, given below; if AB = 8 cm, AD = 10 cm, BD = 12 cm, DC = 13 cm and
DBC = 90o
Solution 8:
9.The base of a triangular field is three times its height. If the cost of cultivating the field at
36.72 per 100 m2 is 49,572; find its base and height.Solution 9:
10.The sides of a triangular field are in the ratio 5 : 3 : 4 and its perimeter is 180 m. Find:
(i) its area.
(ii) altitude of the triangle corresponding to its largest side.
(iii) the cost of leveling the field at the rate of Rs. 10 per square metre.
Solution 10:
11.Each of equal sides of an isosceles triangle is 4 cm greater than its height. IF the base of the triangle is 24 cm; calculate the perimeter and the area of the triangle.
12.Calculate the area and the height of an equilateral triangle whose perimeter is 60 cm.
Solution 12:
13.In triangle ABC; angle A = 90o, side AB = x cm, AC = (x + 5) cm and area = 150 cm2. Find the sides of the triangle.
Solution 13:
14.If the difference between the sides of a right angled triangle is 3 cm and its area is 54 cm2; find its perimeter.
Solution 14:
15.AD is altitude of an isosceles triangle ABC in which AB = AC = 30 cm and BC = 36 cm. A point O is marked on AD in such a way that
BOC = 90o. Find the area of quadrilateral ABOC.Solution 15:
Exercise 20(B)
1.Find the area of a quadrilateral one of whose diagonals is 30 cm long and the perpendiculars from the other two vertices are 19 cm and 11 cm respectively.
Solution 1:
2.The diagonals of a quadrilateral are 16 cm and 13 cm. If they intersect each other at right angles; find the area of the quadrilateral.
Solution 2:
3.Calculate the area of quadrilateral ABCD, in which
ABD = 90o, triangle BCD is an equilateral triangle of side 24 cm and AD = 26 cm.Solution 3:
4.Calculate the area of quadrilateral ABCD in which AB = 32 cm, AD = 24 cm
A = 90o and BC = CD = 52 cm.Solution 4:
5.The perimeter of a rectangular field is
km. If the length of the field is twice its width; find the area of the rectangle in sq. metres.Solution 5:
6.A rectangular plot 85 m long and 60 m broad is to be covered with grass leaving 5 m all around. Find the area to be laid with grass.
Solution 6:
7.The length and the breadth of a rectangle are 6 cm and 4 cm respectively. Find the height of a triangle whose base is 6 cm and area is 3 times that of the rectangle.
Solution 7:
8.How many tiles, each of area 400 cm2, will be needed to pave a footpath which is 2 m wide and surrounds a grass plot 25 m long and 13 m wide?
Solution 8:
9.The cost of enclosing a rectangular garden with a fence all round, at the rate of 75 paise per metre, is Rs. 300. If the length of the garden is 120 metres, find the area of the field in square metres.
Solution 9:
10.The width of a rectangular room is
of its length, x, and its perimeter is y. Write an equation connecting x and y. Find the length of the room when the perimeter is 4400 cm.Solution 10:
11.The length of a rectangular verandah is 3 m more than its breadth. The numerical value of its area is equal to the numerical value of its perimeter.
(i) Taking x as the breadth of the verandah, write an equation in x that represents the above statement.
(ii) Solve the equation obtained in (i) above and hence find the dimensions of the verandah.
Solution 11:
12.The diagram, given below, shows two paths drawn inside a rectangular field 80 m long and 45 m wide. The widths of the two paths are 8 m and 15 m as shown. Find the area of the shaded portion.
_SHR_files/20141014160129_image002.jpg)
Solution 12:
13.The rate for a 1.20 m wide carpet is Rs. 40 per metre; find the cost of covering a hall 45 m long and 32 m wide with this carpet. Also, find the cost of carpeting the same hall if the carpet, 80 wide, is at Rs. 25. Per metre.
We need to find the cost of carpeting of 80 cm = 0.8 m wide carpet, if the rate of carpeting is Rs. 25. Per metre.
Then
14.Find the area and perimeter of a square plot of land, the length of whose diagonal is 15 metres. Given your answer correct to 2 places of decimals.
Solution 14:
15.The shaded region of the given diagram represents the lawn in the form of a house. On the three sides of the lawn there are flowerbeds having a uniform width of 2 m.
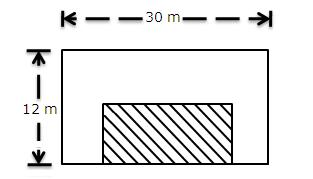
(i) Find the length and the breadth of the lawn.
(ii) Hence, or otherwise, find the area of the flower-beds.
Solution 15:
16.A floor which measures 15 m
8 m is to be laid with tiles measuring 50 m 25 cm. Find the number of tiles required.
Further, if a carpet is laid on the floor so that a space of 1 m exists between its edges and the edges of the floor, what fraction of the floor is uncovered?
17.Two adjacent sides of parallelogram are 24 cm and 18 cm. If the distance between the longer sides is 12 cm; find the distance between the shorter sides.
Solution 17:
18.
Solution 18:
19.The area of a rhombus is 216 sq. cm. If its one diagonals is 24 cm; find:
(i) Length of its other diagonal,
(ii) Length of its side,
(iii) Perimeter of the rhombus.
Solution 19:
20.The perimeter of a rhombus is 52 cm. If one diagonal is 24 cm; find:
(i) The length of its other diagonal,
(ii) Its area.
Solution 20:
21.The perimeter of a rhombus is 46 cm. If the height of the rhombus is 8 cm; find its area.
Solution 21:
22.The figure given below shows the cross-section of a concrete structure. Calculate the area of cross-section if AB = 1.8 cm, CD = 0.6 m, DE = 0.8 m, EF = 0.3 m and AF = 1.2 m.
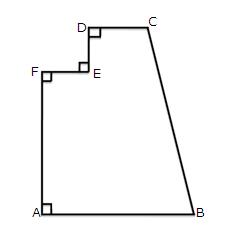
Solution 22:
23.Calculate the area of the figure given below: which is not drawn scale.
Solution 23:
24.The following diagram shows a pentagonal field ABCDE in which the lengths of AF, FG, GH and HD are 50 m, 40 m, 15 m and 25 m respectively; and the lengths of perpendiculars BF, CH and EG are 50 m, 25 m ad 60 m respectively. Determine the area of the field.
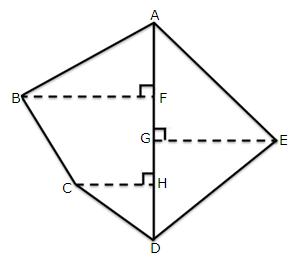
Solution 24:
25.A footpath of uniform width runs all around the outside of a rectangular field 30 m long and 24 m wide. If the path occupies an area of 360 m2, find its width.
Solution 25:
26.A wire when bent in the form of a square encloses an area of 484 m2. Find the largest area enclosed by the same wire when bent to from:
(i) An equilateral triangle.
(ii) A rectangle of length 16 m.
Solution 26:
27.For each trapezium given below; find its area.
(i)
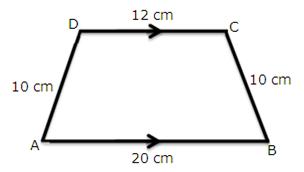
(ii)
(iii)
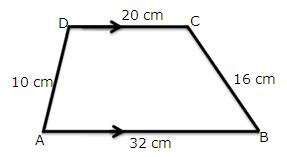
(iv)
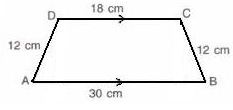
Solution 27:
(iv) In the given figure, we can observe that the non-parallel sides are equal and hence it is an isosceles trapezium.
28.The perimeter of a rectangular board is 70 cm. Taking its length as x cm, find its width in terms of x.
If the area of the rectangular board is 300 cm2; find its dimensions.
Solution 28:
29.The area of a rectangular is 640 m2. Taking its length as x cm; find in terms of x, the width of the rectangle. If the perimeter of the rectangle is 104 m; find its dimensions.
Solution 29:
30.The length of a rectangle is twice the side of a square and its width is 6 cm greater than the side of the square. If area of the rectangle is three times the area of the square; find the dimensions of each.
Solution 30:
31.ABCD is a square with each side 12 cm. P is a point on BC such that area of
ABP: area of trapeziumAPCD = 1: 5. Find the length of CP.Solution 31:
32.A rectangular plot of land measures 45 m
30 m. A boundary wall of height 2.4 m is built all around the plot at a distance of 1 m from the plot. Find the area of the inner surface of the boundary wall.
33.A wire when bent in the form of a square encloses an area = 576 cm2. Find the largest area enclosed by the same wire when bent to form;
(i) an equilateral triangle.
(ii) A rectangle whose adjacent sides differ by 4 cm.
Solution 33:
34.The area of a parallelogram is y cm2 and its height is h cm. The base of another parallelogram is x cm more than the base of the first parallelogram and its area is twice the area of the first. Find, in terms of y, h and x, the expression for the height of the second parallelogram.
Solution 34:
35.The distance between parallel sides of a trapezium is 15 cm and the length of the line segment joining the mid-points of its non-parallel sides is 26 cm. Find the area of the trapezium.
Solution 35:
36.The diagonal of a rectangular plot is 34 m and its perimeter is 92 m. Find its area.
Solution 36:
Exercise 20(C)
1.
Solution 1:
2.The circumference of a circular field is 308 m. Find is:
(i) Radius
(ii) Area.
Solution 2:
3.The sum of the circumference and diameter of a circle is 116 cm. Find its radius.
Solution 3:
4.The radii of two circles are 25 cm and 18 cm. Find the radius of the circle which has circumference equal to the sum of circumferences of these two circles.
Solution 4:
5.The radii of two circles are 48 cm and 13 cm. Find the area of the circle which has its circumference equal to the difference of the circumferences of the given two circles.
Solution 5:
6.The diameters of two circles are 32 cm and 24 cm. Find the radius of the circle having its area equal to sum of the areas of the two given circle.
Solution 6:
7.The radius of a circle is 5 m. Find the circumference of the circle whose area is 49 times the area of the given circle.
Solution 7:
8.A circle of largest area is cut from a rectangular piece of card-board with dimensions 55 cm and 42 cm. Find the ratio between the area of the circle cut and the area of the remaining card-board.
Solution 8:
9.The following figure shows a square cardboard ABCD of side 28 cm. Four identical circles of largest possible size are cut from this card as shown below.
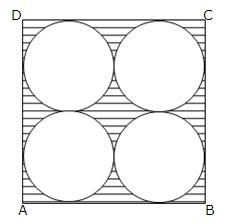
Find the area of the remaining card-board.
Solution 9:
10.The radii of two circles are in the ratio 3 : 8. If the difference between their areas is 2695
cm2, find the area of the smaller circle.Solution 10:
11.The diameters of three circles are in the ratio 3 : 5 : 6. If the sum of the circumferences of these circles be 308 cm; find the difference between the areas of the largest and the smallest of these circles.
Solution 11:
12.Find the area of a ring shaped region enclosed between two concentric circles of radii 20 cm and 15 cm.
Solution 12:
13.The circumference of a given circular park is 55 m. It is surrounded by a path of uniform width 3.5 m . Find the area of the path.
Solution 13:
14.There are two circular gardens A and B. The circumference of garden A is 1.760 km and the area of garden B is 25 times the area of garden A. Find the circumference of garden B.
Solution 14:
15.A wheel has diameter 84 cm. Find how many completer revolutions must it make to cover 3.168 km.
Solution 15:
16.Each wheel of a car is of diameter 80 cm. How many completer revolutions does each wheel make in 10 minutes when the car is travelling at a speed of 66 km per hour?
Solution 16:
17.An express train is running between two stations with a uniform speed. If the diameter of each wheel of the train is 42 cm and each wheel makes 1200 revolutions per minute, find the speed of the train.
Solution 17:
18.The minute hand of a clock is 8 cm long. Find the area swept by the minute hand between 8.30 a.m. and 9.05 a.m.
Solution 18:
19.The shaded portion of the figure, given alongside, shows two concentric circles.
If the circumference of the two circles be 396 cm and 374 cm, find the area of the shaded portion.
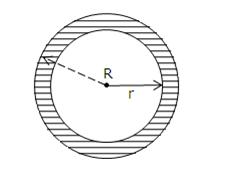
Solution 19:
20.In the given figure, the area of the shaded portion is 770 cm2. If the circumference of the outer circle is 132 cm, find the width of the shaded portion.
Solution 20:
From the given data, we can calculate the area of the outer circle and then the area of inner circle and hence the width of the shaded portion.
21.The cost of fencing a circular field at the rate of
240 per meter is 52,800. The field is to be ploughed at the rate of 12.50 per m2. Find the cost of pouching the field.Solution 21:
22.Two circles touch each other externally. The sum of their areas is 58Selina Solutions Icse Class 9 Mathematics Chapter - Area And Perimeter Of Plane Figures cm2 and the distance between their centers is 10 cm. Find the radii of the two circles.
Solution 22:
23.The given figures shows a rectangle ABCD inscribed in a circle as shown alongside.
If AB = 28 cm and BC = 21 cm, find the area of the shaded portion of the given figure.
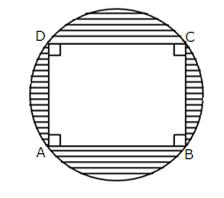
Solution 23:
24.A square is inscribed in a circle of radius 7 cm. Find the area of the square.
Solution 24:
25.
Solution 25:
26.
Solution 26:
27.Two circles touch each other externally. The sum of their areas is 74π cm2 and the distance between their centres is 12 cm. Find the diameters of the circle.
Solution 27:
28.If a square in inscribed in a circle, find the ratio of the areas of the circle and the square.
Solution 28:
Selina Concise Mathematics Class 9 ICSE Maths Solutions Chapter 20 - Area and Perimeter of Plane Figures