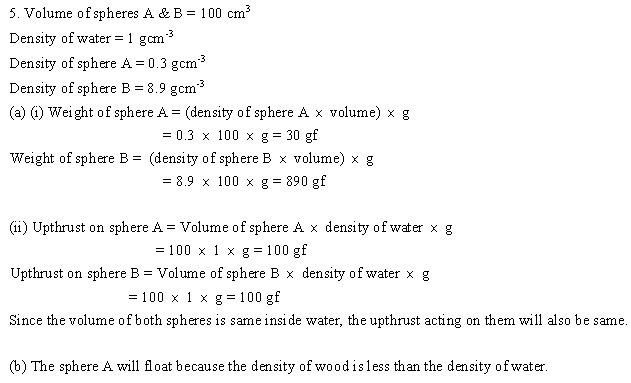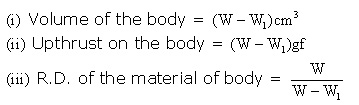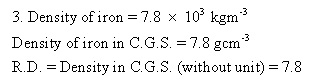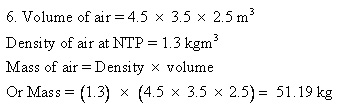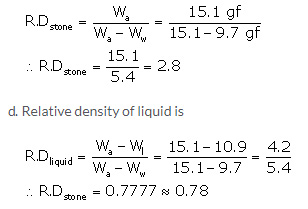Selina ICSE Solutions for Class 9 Physics Chapter 5 Upthrust in Fluids, Archimedes’ Principle and Floatation
Selina ICSE Solutions for Class 9 Physics Chapter 5 Upthrust in Fluids, Archimedes’ Principle and Floatation
Exercise 5(A)1S.What do you understand by the term upthrust of a fluid? Describe an experiment to show its existence.
Solution 1S.
When a body is partially or wholly immersed in a liquid, an upward force acts on it. This upward force is known as an upthrust.
Upthrust can be demonstrated by the following experiment:
Take an empty can and close its mouth with an airtight stopper. Put it in a tub filled with water. It floats with a large part of it above the surface of water and only a small part of it below the surface of water. Push the can into the water. You can feel an upward force and you find it difficult to push the can further into water. It is noticed that as the can is pushed more and more into the water, more and more force is needed to push the can further into water, until it is completely immersed. When the can is fully inside the water, a definite force is still needed to keep it at rest in that position. Again, if the can is released in this position, it is noticed that the can bounces back to the surface and starts floating again.
2S.In what direction and at what point does the buoyant force on a body due to a liquid act?
Solution 2S.
Buoyant force on a body due to a liquid acts upwards at the centre of buoyancy.
3S.What is meant by the term buoyancy?
Solution 3S.
The property of a liquid to exert an upward force on a body immersed in it is called buoyancy.
4S.Define upthrust and state its S.I. unit.
Solution 4S.
The upward force exerted on a body by the fluid in which it is submerged is called the upthrust. Its S.I. unit is ‘newton’.
5S.What is the cause of upthrust? At which point can it be considered to act?
Solution 5S.
A liquid contained in a vessel exerts pressure at all points and in all directions. The pressure at a point in a liquid is the same in all directions – upwards, downwards and sideways. It increases with the depth inside the liquid.
When a body is immersed in a liquid, the thrusts acting on the side walls of the body are neutralized as they are equal in magnitude and opposite in direction. However, the magnitudes of pressure on the upper and lower faces are not equal. The difference in pressure on the upper and lower faces cause a net upward force (= pressure x area) or upthrust on the body.
It acts at the centre of buoyancy.
6S.Why is a force needed to keep a block of wood inside water?
Solution 6S.
Upthrust due to water on block when fully submerged is more than its weight. Density of water is more than the density of cork; hence, upthrust due to water on the block of cork when fully submerged in water is more than its weight.
7S.A piece of wood if left under water comes to the surface. Explain the reason.
Solution 7S.
A piece of wood if left under water comes to the surface of water because the upthrust on body due to its submerged part is equal to its own weight.
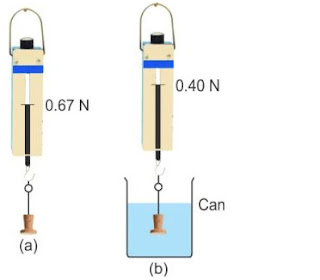
8S.Describe an experiment to show that a body immersed in a liquid appears lighter than it really is.
Solution 8S.
Experiment to show that a body immersed in a liquid appears lighter:
Take a solid body and suspend it by a thin thread from the hook of a spring balance as shown in the above figure (a). Note its weight. Above figure (a) shows the weight as 0.67 N.
Then, take a can filled with water. Immerse the solid gently into the water while hanging from the hook of the spring balance as shown in figure (b). Note its weight. Above figure (b) shows the weight as 0.40 N.
The reading in this case (b) shall be less than the reading in the case (a), which proves that a body immersed in a liquid appears to be lighter.
9S.A metal solid cylinder tied to a thread is hanging from the hook of a spring balance. The cylinder is gradually immersed into the water contained in a jar. What changes do you expect in the readings of the spring balance? Explain your answer.
Solution 9S.
The readings in the spring balance decreases.
As the cylinder is immersed in the jar of water, an upward force acts on it, which is in opposition to the weight component of the cylinder. Hence the cylinder appears to be lighter.
10S.Will a body weigh more in air or vacuum when weighed with a spring balance? Give a reason for your answer.
Solution 10S.
A body shall weigh more in vacuum because in vacuum, i.e. in absence of air, no upthrust will act on the body.
11S.A body dipped into a liquid experiences an upthrust. State two factors on which upthrust on the body depends.
Solution 11S.
Upthrust on a body depends on the following factors:
Volume of the body submerged in the liquid or fluid.
Density of liquid or fluid in which the body is submerged.
12S.How is the upthrust related to the volume of the body submerged in a liquid?
Solution 12S.
Larger the volume of body submerged in liquid, greater is the upthrust acting on it.
13S.
Solution 13S.
A stone falls faster.
Because the volume of stone is less than the volume of bunch of feathers of the same mass, the upthrust due to air on stone is less than that on the bunch of feathers, and hence, the stone falls faster.
However, in vacuum, both shall fall together because there will be no upthrust.
14S.A body experiences an upthrust F1 in river water and F2 in sea water when dipped up to the same level. Which is more, F1 or F2? Give reason.
Solution 14S.
F2 > F1; Sea water is denser than river water; therefore, the upthrust due to sea water will be greater than that due to river water at the same level. This shall make the body to appear lighter in the sea water.
15S.A small block of wood is completely immersed in (i) water, (ii) glycerine and then released. In each case, What do you observe? Explain the difference in your observation in the two cases.
Solution 15S.
Observation: Volume of a block of wood immersed in glycerine is smaller as compared to the volume of block immersed in water.
Explanation: Density of glycerine is more than that of water. Hence, glycerine exerts more upthrust on the block of wood than water, causing it to float in glycerine with a smaller volume.
16S.A body of volume V and density p is kept completely immersed in a liquid of density pl. If g is the acceleration due to gravity, then write expressions for the following:
(i) The weight of the body, (ii) The upthrust on the body,
(iii) The apparent weight of the body in liquid, (iv) The loss in weight of the body.
Solution 16S.
17S.A body held completely immersed inside a liquid experiences two forces:
(i) F1, the force due to gravity and
(ii) F2, the buoyant force.
Draw a diagram showing the direction of these forces acting on the body and state the condition when the body will float or sink.
Solution 17S.
18S.Complete the following sentences:
(a) Two balls, one of iron and the other of aluminium experience the same upthrust when dipped completely in water if _____________ .
(b) An empty tin container with its mouth closed has an average density equal to that of a liquid. The container is taken 2 m below the surface of that liquid and is left there. Then the container will ____________ .
(c) A piece of wood is held under water. The upthrust on it will be
___________ the weight of the wood piece.
Solution 18S.
(a) Both have equal volumes.
(b) Bounce back to the surface.
(c) More than
19S.Prove that the loss in weight of a body when immersed wholly or partially in a liquid is equal to the buoyant force (or upthrust) and this loss is because of the difference in pressure exerted by liquid on the upper and lower surfaces of the submerged part of body.
Solution 19S.
Consider a cylindrical body PQRS of cross-sectional area A immersed in a liquid of density ρ as shown in the figure above. Let the upper surface PQ of the body is at a depth h1 while its lower surface RS is at depth h2 below the free surface of liquid.
At depth h1, the pressure on the upper surface PQ,
P1 = h1 ρg.
Therefore, the downward thrust on the upper surface PQ,
F1 = Pressure x Area = h1 ρgA ……………….(i)
At depth h2, pressure on the lower surface RS,
P2 = h2 ρg
Therefore, the upward thrust on the lower surface RS,
F2 = Pressure x Area = h2 ρgA …………………(ii)
The horizontal thrust at various points on the vertical sides of body get balanced because the liquid pressure is the same at all points at the same depth.
From the above equations (i) and (ii), it is clear that F2 > F1 because h2 > h1 and therefore, body will experience a net upward force.
Resultant upward thrust or buoyant force on the body,
FB = F2 – F1
= h2 ρgA – h1 ρgA
= A (h2 – h1) ρg
However, A (h2 – h1) = V, the volume of the body is submerged in a liquid.
Therefore, upthrust FB = V ρg.
Now, V g = Volume of solid immersed x Density of liquid x Acceleration due to gravity
= Volume of liquid displaced x Density of liquid x Acceleration due to gravity
= Mass of liquid displaced x Acceleration due to gravity
= Weight of the liquid displaced by the submerged part of the body
Thus, Upthrust FB = weight of the liquid displaced by the submerged part of the body…..(iii)
Now, let us take a solid and suspend it by a thin thread from the hook of a spring balance and note its weight.
Then take a eureka can and fill it with water up to its spout. Arrange a measuring cylinder below the spout of the eureka can as shown. Immerse the solid gently in water. The water
displaced by the solid is collected in the measuring cylinder.
When the water stops dripping through the spout, note the weight of the solid and volume of water collected in the measuring cylinder.
From the diagram, it is clear that
Loss in weight (Weight in air – Weight in water) = Volume of water displaced.
Or, Loss in weight = Volume of water displaced x 1 gcm-3 [Because the density of water = 1 gcm-3]
Or, Loss in weight = Weight of water displaced ……………(iv)
From equations (iii) and (iv),
Loss in weight = Upthrust or buoyant force
20S.A sphere of iron and another sphere of wood of the same radius are held under water. Compare the upthrust on the two spheres.
Hint: Both have equal volume inside the water.
Solution 20S.
Since the spheres have the same radius, both will have an equal volume inside water, and hence, the upthrust acted by water on both the spheres will be the same.
Hence, the required ratio of upthrust acting on two spheres is 1:1.
21S.A sphere of iron and another of wood, both of same radius are placed on the surface of water. State which of the two will sink? Give a reason for your answer.
Solution 21S.
Sphere of iron will sink.
Density of iron is more than the density of water, so the weight of iron sphere will be more than the upthrust due to water in it; thus, it causes the iron sphere to sink.
Density of wood is less than the density of water, so the weight of sphere of wood shall be less than the upthrust due to water in it. So, the sphere of wood will float with a volume submerged inside water which is balanced by the upthrust due to water.
22S.How does the density of material of a body determine whether it will float or sink in water?
Solution 22S.
The bodies of average density greater than that of the liquid sink in it. While the bodies of average density equal to or smaller than that of liquid float on it.
23S.A body of density p is immersed in a liquid of density pl .State the condition when the body will (i) float and (ii) sink in the liquid.
Solution 23S.
(i) The body will float if ρ ≤ ρL
(ii) The body will sink if ρ > ρL
24S.It is easier to lift a heavy stone under water than in air. Explain.
Solution 24S.
It is easier to lift a heavy stone under water than in air because in water, it experiences an upward buoyant force which balances the actual weight of the stone acting downwards. Thus, due to upthrust there is an apparent loss in the weight of the heavy stone, which makes it lighter in water, and hence easy to lift.
25S.State the Archimedes' principle.
Solution 25S.
Archimedes’ principle states that when a body is immersed partially or completely in a liquid, it experiences an upthrust, which is equal to the weight of liquid displaced by it.
26S.Describe an experiment to verify the Archimedes' principle.
Solution 26S.
Let us take a solid and suspend it by a thin thread from the hook of a spring balance and note its weight (Fig a).
Then take a eureka can and fill it with water up to its spout. Arrange a measuring cylinder below the spout of the eureka can as shown. Immerse the solid gently in water. The water displaced by the solid gets collected in the measuring cylinder.
When water stops dripping through the spout, note the weight of the solid and volume of water collected in the measuring cylinder.
From diagram, it is clear that
Loss in weight (Weight in air – weight in water) = 300 gf – 200 gf = 100 gf
Volume of water displaced = Volume of solid = 100 cm3
Because density of water = 1 gcm-3
Weight of water displaced = 100 gf = Upthrust or loss in weight
This verifies Archimedes’ principle.
1M.A body will experience minimum upthrust when it is completely immersed in which of the following liquids:
(a) Turpentine (b) Water
(c) Glycerine (d) Mercury
Solution 1M.
Turpentine
2M.The S.I. unit of upthrust is given by the following unit:
(a) Pa (b) N
(c) kg (d) kg m2
Solution 2M.
N
3M.A body of density
(a) 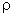 =
= 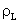 (b)
(b) 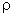
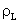
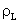 (b)
(b) 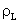
(c) 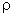
 (d) Nothing can be said.
(d) Nothing can be said.
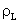 (d) Nothing can be said.
(d) Nothing can be said.
Solution 3M.
ρ > ρL
1N.A body of volume 100 cm3 weighs 5 kgf in air. It is completely immersed in a liquid of density 1.8 x 103 kg m-3. Find:
(i) The upthrust due to liquid and
(ii) The weight of the body in liquid.
(i) The upthrust due to liquid and
(ii) The weight of the body in liquid.
Solution 1N.
2N.A body weighs 450 gf in air and 310 gf when completely immersed in water. Find the following factors:
(i) The volume of the body,
(ii) The loss in weight of the body, and (iii) The upthrust on the body.
State the assumption made in part (i).
(i) The volume of the body,
(ii) The loss in weight of the body, and (iii) The upthrust on the body.
State the assumption made in part (i).
Solution 2N.
3N.You are provided with a hollow iron ball A of volume 15 cm3 and mass 12 g and a solid iron ball B of mass 12 g. Both are placed on the surface of water contained in a large tub.
(a) Find upthrust on each ball.
(b) Which ball will sink? Give a reason for your answer. (Density of iron = 8.0 g cm-3)
(a) Find upthrust on each ball.
(b) Which ball will sink? Give a reason for your answer. (Density of iron = 8.0 g cm-3)
Solution 3N.
4N.A solid of density 5000 kg m-3 weighs 0.5 kgf in air. It is completely immersed in water of density 1000 kg m-3. Calculate the apparent weight of the solid in water.
Solution 4N.
5N.Two spheres A and B, each of volume 100 cm3 are placed on water (density = 1.0 g cm-3). The sphere A is made of wood of density 0.3 g cm-3 and the sphere B is made of iron of density 8.9 g cm-3.
(a) Find:
(i) The weight of each sphere, and
(ii) The upthrust on each sphere.
(b) Which sphere will float? Give reason.
(a) Find:
(i) The weight of each sphere, and
(ii) The upthrust on each sphere.
(b) Which sphere will float? Give reason.
Solution 5N.
6N.The mass of a block made of certain material is 13.5 kg and its volume is 15 × 10-3 m3.
(a) Calculate upthrust on the block if it is held fully immersed in water.
(b) Will the block float or sink in water when released? Give a reason for your answer.
(c) What will be the upthrust on block while floating?
Take density of water = 1000 kg m-3.
(a) Calculate upthrust on the block if it is held fully immersed in water.
(b) Will the block float or sink in water when released? Give a reason for your answer.
(c) What will be the upthrust on block while floating?
Take density of water = 1000 kg m-3.
Solution 6N.
7N.A piece of brass weighs 175 gf in air and 150 gf when fully submerged in water. The density of water is 1.0 g cm3.
(i) What is the volume of the brass piece? (ii) Why does the brass piece weigh less in water?
Solution 7N.
8N.A metal cube of edge 5 cm and density 9 g cm-3 is suspended by a thread so as to be completely immersed in a liquid of density 1.2 g cm-3. Find the tension in thread. (Take g = 10 m s-2)
Solution 8N.
9N.A block of wood is floating on water with its dimensions 50 cm x 50 cm x 50 cm inside water. Calculate the buoyant force acting on the block. Take g = 9.8 N kg-1.
Solution 9N.
10N.A body of mass 3.5 kg displaces 1000 cm3 of water when fully immersed inside it. Calculate: (i) the volume of body, (ii) the upthrust on body and (iii) the apparent weight of body in water.
Solution 10N.
Exercise 5(B)
1S.Define the term density.
Solution 1S.
The density of a substance is its mass per unit volume.
2S.State (i) C.G.S. and (ii) S.I. units of density.
Solution 2S.
(i) The C.G.S. unit of density is gcm-3.
(ii) The S.I. unit of density is kgm-3.
3S.Express the relationship between the C.G.S. and S.I. units of density.
Solution 3S.
1 gcm-3 = 1000 kgm-3
4S.'The density of iron is 7800 kg m-3'. What do you understand by this statement?
Solution 4S.
It means the mass of 1 m-3 of iron is 7800 kg.
5S.Write the density of water at 4°C in S.I. units.
Solution 5S.
Density of water at 4°C in S.I. units is 1000 kgm-3.
6S.How are the (i) Mass, (ii) Volume and (iii) Density of a metallic piece affected, if at all, with an increase in temperature?
Solution 6S.
(i) Mass of a metallic body remains unchanged with increase in temperature.
(ii) Volume of metallic body increases with an increase in temperature.
(iii) Density (= Mass/volume) of a metallic body decreases with an increase in temperature.
7S.Water is heated from 0°C to 10°C . How does the density of water change with temperature?
Solution 7S.
On heating from 0°C, the density of water increases up to 4°C and then decreases beyond 4°C.
8S.Complete the following sentences.
(i) Mass = .................... × density
(ii) S.I. unit of density is .......... .
(iii) Density of water is .... ... kg m-3.
(iv) Density in kg m-3 = ............ × density in g cm-3
Solution 8S.
(i) Volume, (ii) kg m-3, (iii) 1000 and (iv) 1000
9S.What do you understand by the term relative density of a substance?
Solution 9S.
The relative density of a substance is the ratio of density of that substance to the density of water at 4°C.
10S.What is the unit of relative density?
Solution 10S.
Relative density is the ratio of two similar quantities; thus, it has no unit.
11S.Differentiate between density and relative density of a substance.
Solution 11S.
Density of a substance is the ratio of its mass to its volume but R.D. of a substance is the ratio of density of that substance to the density of water at 4°C.
12S.With the use of Archimedes' principle, state how you will find relative density of a solid denser than water. How will you modify your experiments if the solid is soluble in water?
Solution 12S.
Steps:
With the help of a physical balance, find the weight, W1 of the given solid.
Immerse the solid completely in a beaker filled with water such that it does not touch the walls and bottom of beaker, and find the weight W2 of solid in water.
Observations:
Loss in weight of solid when immersed in water = (W1 – W2) gf
R.D. = Weight of solid in air/Loss of weight of solid in water
R.D. = W1/(W1 – W2).
If the solid is soluble in water, then instead of water, take a liquid in which the solid is insoluble and it sinks in the liquid.
Then, R.D. = (Weight of solid in air/Loss of weight of solid in liquid) x R.D. of the liquid
13S.A body weighs W gf in air and W1 gf when it is completely immersed in water. Find: (i) Volume of the body, (ii) Upthrust on the body and (iii) Relative density of the material of the body.
Solution 13S.
14S.
Solution 14S.
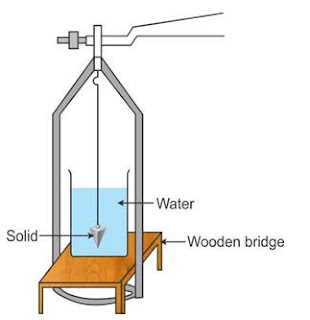
Experimental determination of R.D. of a solid lighter than water (such as cork):
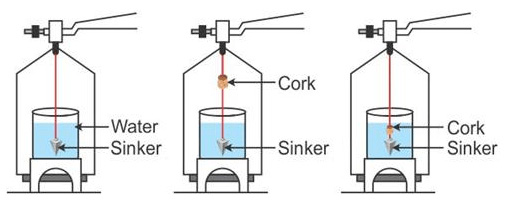
- Take a sinker (i.e. a piece of metal or stone).
- Place a beaker nearly two-third filled with water on a wooden bridge kept over the left pan of a physical balance.
- Suspend the sinker with a thread from the hook of the left pan of balance so that it is completely immersed in water (as shown in the figure below). Find the weight W1 of the sinker in water.
- Tie the given solid (say, a cork) in the middle of a thread, and then measure the weight W2 of a solid in the air along with the sinker in water.
- Tie the cork with the sinker and immerse both of them completely in water of beaker and measure the weight W3 of the solid and sinker both in water.
Observations:
Weight of the sinker in water = W1gf
Weight of the sinker in water and cork in air = W2gf
Weight of sinker and cork together in water = W3gf
Calculations:
Weight of cork in air = (W2 – W1) gf
Weight of cork in water = (W3 – W1) gf
Loss in weight of the cork in water = Weight of cork in air Weight of cork in water.
= [(W2 – W1) – (W3 – W1)] gf
= (W2 – W3) gf
R.D. of cork = Weight of cork in air/Loss of weight of cork in water
Or, R.D. of cork = (W2 – W1)/(W2 – W3).
15S.
Solution 15S.
The weight of the sinker and cork combined, in water will be less than the weight of the sinker alone in water because the upthrust due to water on cork (when completely immersed) is more than the weight of cork itself.
1M.Relative density of a substance is expressed by comparing the density of that substance with the density of the substance listed below:
(a) Air (b) Mercury (c) Water (d) Iron
(a) Air (b) Mercury (c) Water (d) Iron
Solution 1M.
Water
2M.The unit of relative density is which of the following listed below:
(a) g cm-3 (b) kg m-3 (c) m3 kg-1 (d) no unit
Solution 2M.
No unit.
3M.The density of water is as listed below:
(a) 1000 g cm-3 (b) 1 kg m-3 (c) 1 g cm-3 (d) None of these.
Solution 3M.
1 g cm-3
1N.The density of copper is 8.83 g cm-3. Express it in kg m-3.
Solution 1N.
2N.The relative density of mercury is 13.6. State its density in (i) C.G.S. unit and (ii) S.I. unit.
Solution 2N.
3N.The density of iron is 7.8 x 103 kg m-3. What is its relative density?
Solution 3N.
4N.The relative density of silver is 10.8. Find its density.
Solution 4N.
5N.Calculate the mass of a body whose volume is 2 m3 and relative density is 0.52.
Solution 5N.
6N.Calculate the mass of air in a room of dimensions 4.5 m × 3.5 m × 2.5 m if the density of air at N.T.P. is 1.3 kgm-3 .
Solution 6N.
7N.A piece of stone of mass 113 g sinks to the bottom in water contained in a measuring cylinder and water level in cylinder rises from 30 ml to 40 ml. Calculate R.D. of stone.
Solution 7N.
8N.A body of volume 100 cm3 weighs 1 kgf in air. Find: (i) Its weight in water and (ii) Its relative density.
Solution 8N.
9N.A body of mass 70 kg, when completely immersed in water, displaces 20,000 cm3 of water. Find: (i) The mass of body in water and (ii) The relative density of material of the body.
Solution 9N.
10N.A solid weighs 120 gf in air and and 105 gf when it is completely immersed in water. Calculate the relative density of solid.
Solution 10N.
11N.A solid weighs 32 gf in air and 28.8 gf in water. Find: (i) The volume of solid, (ii) R.D. of solid and (iii) The weight of solid in a liquid of density 0.9 g cm-3.
Solution 11N.
12N.A body weighs 20 gf in air and 18.0 gf in water. Calculate the relative density of the material of the body.
Solution 12N.
13N.A solid weighs 1.5 kgf in air and 0.9 kgf in a liquid of density 1.2 x 103 kg m-3. Calculate R.D. of solid.
Solution 13N.
14N.A jeweller claims that he makes ornaments of pure gold that has a relative density of 19.3. He sells a bangle weighing 25.25 gf to a person. The clever customer weighs the bangle when immersed in water and finds that it weighs 23.075 gf in water. With the help of suitable calculations, find out whether the ornament is made of pure gold or not.
Hint : Calculate R.D. of the material of the bangle.
Solution 14N.
15N.
Solution 15N.
16N.A piece of stone of mass 15.1 g is first immersed in a liquid and it weighs 10.9 gf. Then on immersing the piece of stone in water, it weighs 9.7 gf. Calculate:
a. The weight of the piece of stone in air,
b. The volume of the piece of stone,
c. The relative density of stone,
d. The relative density of the liquid.
Solution 16N.
a. The mass of stone is 15.1 g. Hence, its weight in air will be Wa = 15.1 gf
b. When stone is immersed in water its weight becomes 9.7 gf. So, the upthrust on the stone is 15.1 – 9.7 = 5.4 gf, Since the density of water is 1 g cm-3, the volume of stone is 5.4 cm3.
c. Weight of stone in liquid is Wl = 10.9 gf
Weight of stone in water is Ww = 9.7 gf
Therefore, the relative density of stone is
Exercise 5(C)
1S.State the principle of floatation.
Solution 1S.
According to the principle of floatation, the weight of a floating body is equal to the weight of the liquid displaced by its submerged part.
2S.A body is held immersed in a liquid. (i) Name the two forces acting on the body and draw a diagram to show these forces. (ii) State how the magnitudes of two forces mentioned in part (i) determine whether the body will float or sink in liquid when it is released. (iii) What is the net force on the body if it (a) sinks and (b) floats?
Solution 2S.
(i) Two forces acting on the body are as listed below:
(a) Weight of the body (downwards)
(b) Upthrust of the liquid (upwards)
(ii) If the weight of the body is greater than the upthrust acting on it, the body will sink
If the weight of the body is equal to or less than the upthrust acting on it, the body will float.
(iii) (a) The net force acting on the body when it sinks is body’s own weight.
(b) The net force acting on the body when it floats is the upthrust due to the liquid.
3S.When a piece of wood is suspended from the hook of a spring balance, it reads 70 gf. The wood is now lowered into water. What reading do you expect on the scale of spring balance?
Solution 3S.
The reading on the spring balance will be zero because wood floats on water and while floating the apparent weight = 0.
4S.A solid iron ball of mass 500 g is dropped in mercury contained in a beaker. (a) Will the ball float or sink? Give reasons. (b) What will be the apparent weight of the ball?
Solution 4S.
(a) The ball will float because the density of ball (i.e. iron) is less than the density of mercury.
(b) While floating, the apparent weight = 0.
5S.How does the density of a substance determine whether a solid piece of that substance will float or sink in a given liquid?
Solution 5S.
The body will float if its density is less than or equal to the density of the liquid ρS ≤ ρL.
The body will sink if its density is greater than the density of the liquid ρS > ρL.
6S.Explain why an iron nail floats on mercury, but it sinks in water.
Hint: Density of iron is less than that of mercury, but more than that of water.
Solution 6S.
Density of iron is less than the density of mercury; hence, an iron nail floats in mercury and density of iron is more than the density of water; hence, an iron nail sinks in water.
7S.A body floats in a liquid with a part of it submerged inside the liquid. (i) Is the weight of floating body greater than, equal to or less than the upthrust? (ii) What is the apparent weight of the floating body?
Solution 7S.
(i) Weight of the floating body is equal to the upthrust.
(ii) While floating, the apparent weight is zero.
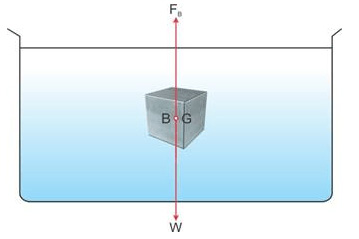
8S.A homogeneous block floats on water (a) partly immersed (b) completely immersed. In each case state the position of centre of buoyancy B with respect to the centre of gravity G of the block.
Solution 8S.
When the body is partially immersed, its centre of buoyancy will be below the centre of gravity of the block.
When the body is completely immersed, its centre of buoyancy will coincide the centre of gravity.
9S.Fig. shows the same block of wood floating in three different liquids A, B and C of densities

Solution 9S.
The upthrust on the body by each liquid is the same and equal to the weight of the body.
However, upthrust = Volume submerged × ρL × g,
For the liquid C, since the volume submerged is least so the density ρ3 must be maximum.
10S.Draw a diagram to show the forces acting on a body floating in water with its some part submerged. Name the forces and show their points of application. How is the weight of water displaced by the floating body related to the weight of the body itself?
Solution 10S.
The forces acting are as listed below:
- Weight of the body acting downwards.
- Upthrust due to water acting upwards.
Weight of water displaced by the floating body = Weight of the body
11S.What is the centre of buoyancy? State its position for a floating body with respect to the centre of gravity of the body.
Solution 11S.
Centre of buoyancy: It is the point through which the resultant of the buoyancy forces on a submerged body act; it coincides with the centre of gravity of the displaced liquid, if the body is completely immersed.
For a floating body with its part submerged in the liquid, the centre of buoyancy is at the centre of gravity of the submerged part of the body and it lies vertically below the centre of gravity of the entire body.
12S.A balloon filled with helium gas floats in a big closed jar which is connected to an evacuating pump. What will be your observation, if air from the jar is pumped out? Explain your answer.
Solution 12S.
Observation : The balloon will sink.
Explanation : As air is pumped out from jar, the density of air in jar decreases, so the upthrust on balloon decreases. As weight of balloon exceeds the upthrust on it, it sinks.
13S.A block of wood is so loaded that it just floats in water at room temperature. What change will occur in the state of floatation, if
(a) Some salt is added to water, (b) Water is heated?
Give reasons.
Solution 13S.
(a) It will float with some part outside water.
Reason : On adding some salt to water, the density of water increases, so upthrust on a block of wood increases, and hence, the block rises up till the weight of salty water displaced by the submerged part of block becomes equal to the weight of the block.
(b) The block will sink.
Reason: On heating, the density of water decreases, so upthrust on the block decreases and the weight of block exceeds upthrust due to which it sinks.
14S.A body of volume V and density
 .
.
Solution 14S.
15S.Why is the floating ice less submerged in brine than in water?
Solution 15S.
Density of brine is more than the density of water. Hence, the upthrust exerted by brine is more than the upthrust exerted by water on ice. Therefore, floating ice is less submerged in brine.
16S.A man first swims in sea water and then in river water. (i) Compare the weights of sea water and river water displaced by him.
(ii) Where does he find it easier to swim and why?
Solution 16S.
(i) 1:1; The weight of the water displaced by the man in sea and river will be same and will be equal to his own weight.
(ii) He finds it easier to swim in the sea because the density of sea water is more than the density of river water. So his weight is balanced in sea water with a part of his body submerged in the water.
17S.An iron nail sinks in the water while an iron ship floats on water. Explain the reason.
Solution 17S.
An iron nail sinks in water because density of iron is more than the density of water, so the weight of the nail is more than the upthrust of water on it.
On the other hand, ships are also made of iron, but they do not sink. This is because the ship is hollow and the empty space in it contains air, which makes its average density less than that of water. Therefore, even with a small portion of ship submerged in water, the weight of water displaced by the submerged part of ship becomes equal to the total weight of ship and it floats.
18S.What can you say about the average density of a ship floating on water in relation to the density of water?
Solution 18S.
Due to the hollow and empty space in the ship, the average density of a ship is less than the density of water.
19S.A piece of ice floating in a glass of water melts, but the level of water in the glass does not change.
Give reasons.
Hint: Ice contracts on melting.
Solution 19S.
When a floating piece of ice melts into water, it contracts by the volume equal to the volume of ice pieces above the water surface while floating on it. Hence, the level of water does not change when ice floating on it melts.
20S.A body is held inside water contained in a vessel by tying it with a thread to the base of the vessel. Name the three forces that keep the body in equilibrium, and state the direction in which each force acts.
Solution 20S.
Forces acting on the body are listed below:
Weight of the body vertically downwards.
Upthrust of water on body vertically upwards.
Tension in thread vertically downwards.
21S.A loaded cargo ship sails from sea water to river water. List your observations.
Solution 21S.
A ship submerges more as it sails from sea water to river water.
Density of river water is less than the density of sea water. Hence, according to the law of floatation, to balance the weight of the ship, a greater volume of water is required to be displaced in river water of lower density.
22S.Explain the following observations listed below:
(a) Icebergs floating in sea are dangerous for ships.
(b) An egg sinks in fresh water, but floats in a strong salt solution.
(c) Toy balloons filled with hydrogen rise to the ceiling, but if they are filled with carbon dioxide, then they sink to the floor.
(d) As a ship in harbour is being unloaded, it slowly rises higher in water.
(e) A balloon filled with hydrogen rises to a certain height and then stops rising further.
(f) A ship submerges more as it sails from sea water to river water.
Solution 22S.
(a) Icebergs are dangerous for ships as they may collide with them. Icebergs being lighter than water, float on water with a major part of their surfaces laying under the water surface and only a small part lies outside water. Thus, it becomes difficult for the driver of the ship to estimate the size of the iceberg.
(b) Density of a strong salt solution is more than the density of fresh water. Hence, the salt solution exerts a greater upthrust on the egg which balances the weight of the egg, so the egg floats in a strong salt solution but sinks in fresh water.
(c) Density of hydrogen is much less than the density of carbon dioxide. When a balloon is filled with hydrogen, the weight of the air displaced by an inflated balloon (i.e. upthrust) becomes more than the weight of a gas filled balloon, and hence, it rises. In case of a balloon filled with carbon dioxide, weight of the balloon becomes more than the upthrust of the air, and hence, it sinks to the floor.
(d) As a ship in harbor is unloaded, its weight decreases. As a result, it displaces less water, and the ship’s hull rises in water till the weight of the water displaced balances the weight of the unloaded ship.
(e) The reason is that the density of air decreases with altitude. Therefore, as the balloon gradually goes up, the weight of the displaced air (i.e. uphrust) decreases. It keeps on rising as long as the upthrust exceeds its weight. When upthrust becomes equal to its weight, it stops rising.
(f) Density of river water is less than the density of sea water. Hence, according to the law of floatation, to balance the weight of the ship, a great volume of water is required to be displaced in river water having a comparitively lower density.
1M.For a floating body, its weight W and upthrust FB on it are related as listed below:
(a) W FB (b) W
FB (b) W FB
FB
(c)W= FB (d)Nothing can be said.
Solution 1M.
W= FB
2M.A body of weight W is floating in a liquid. Its apparent weight will be observed as listed below:
(a) Equal to W (b) Less than W
(c) Greater than W (d) Zero
Solution 2M.
Zero
3M.A body floats in a liquid A of density
(a) 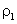 =
= 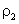 (b)
(b) 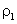

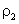
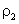 (b)
(b) 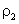
(c) 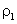

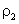 (d) Nothing can be said
(d) Nothing can be said
Solution 3M.
ρ1 > ρ2
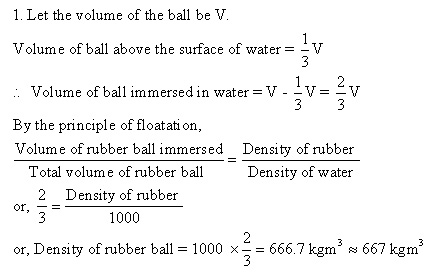
1N.A rubber ball floats on water with its 1/3rd volume outside water. What is the density of rubber?
Solution 1N.
2N.A block of wood of mass 24 kg floats in water. The volume of wood is 0.032 m3. Find the following factors listed below:
(a) The volume of block below the surface of water, (b) The density of wood.
(Density of water = 1000 kg m-3)
Solution 2N.
3N.A wooden cube of side 10 cm has mass 700 g. What part of it remains above the water surface while floating vertically on water surface?
Solution 3N.
4N.A piece of wax floats in brine. What fraction of its volume will be immersed?
Density of wax = 0.95 g cm-3, Density of brine = 1.1 g cm-3.
Density of wax = 0.95 g cm-3, Density of brine = 1.1 g cm-3.
Solution 4N.
5N.If the density of ice is 0.9 g cm-3, then what portion of an iceberg will remain below the surface of water in sea? (Density of sea water = 1.1 g cm-3)
Solution 5N.
6N.A piece of wood of uniform cross section and height 15 cm floats vertically with its height 10 cm in water and 12 cm in spirit. Find the densities of wood and spirit.
Solution 6N.
7N.A wooden block floats in water with two-third of its volume submerged. (a) Calculate the density of wood. (b) When the same block is placed in oil, three-quarters of its volume is immersed in oil. Calculate the density of oil.
Solution 7N.
8N.The density of ice is 0.92 g cm-3 and that of sea water is 1.025 g cm-3. Find the total volume of an iceberg which floats with its volume 800 cm3 above water.
Solution 8N.
9N.A weather forecasting plastic balloon of volume 15 m3 contains hydrogen of density 0.09 kg m-3. The volume of an equipment carried by the balloon is negligible compared to its own volume. The mass of an empty balloon alone is 7.15 kg. The balloon is floating in air of density 1.3 kg m-3.
Calculate: (i) The mass of hydrogen in the balloon, (ii) The mass of hydrogen and balloon, (iii) The total mass of hydrogen, balloon and equipment if the mass of equipment is x kg, (iv) The mass of air displaced by balloon and (v) The mass of equipment using the law of floatation.
Solution 9N.
Selina ICSE Solutions for Class 9 Physics Chapter 5 Upthrust in Fluids, Archimedes’ Principle and Floatation